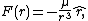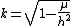“Cotes's spiral”的意思、由来-开放百科全书

In physics and in the mathematics of plane curves, Cotes's spiral (also written Cotes' spiral and Cotes spiral) is a family of spirals named after Roger Cotes.

The shape of spirals in the family depends on the parameters, and the curve equation in polar coordinates can take one of five forms:

A, k and ε are arbitrary real number constants. A determines the size, k determines the shape, and ε determines the angular position of the spiral.

Cotes referred to the different forms as "cases". The curves above correspond to his cases 1, 5, 4, 2, 3 respectively.

The first form is an epispiral; the second is a Poinsot's spiral; the third form is a hyperbolic spiral, which can be seen as the limiting case between an epispiral and a Poinsot's spiral; the fourth is the equiangular spiral.

Classical mechanics

Cotes's spirals appear in classical mechanics, as the family of solutions for the motion of a particle moving under an inverse-cube central force. Consider a central force

where μ is the strength of attraction. Consider a particle moving under the influence of the central force, and let h be its specific angular momentum, then the particle moves along a Cotes's spiral, with the constant k of the spiral given by

when μ > h², Poinsot form of the spiral. When μ = h², the particle follows a hyperbolic spiral. The derivation can be found in the references.^[1]^[2]

History

In the Harmonia Mensurarum (1722), Roger Cotes analysed a number of spirals and other curves, such as the Lituus. He described the possible trajectories of a particle in an inverse-cube central force field, which are the Cotes's spirals. The analysis is based on the method in the Principia Book 1, Proposition 42, where the path of a body is determined under an arbitrary central force, initial speed, and direction.

Depending on the initial speed and direction he determines that there are 5 different "cases" (excluding the trivial ones, the circle and straight line through the centre).

He notes that of the 5, "the first and the last are described by Newton, by means of the quadrature (i.e. integration) of the hyperbola and the ellipse".

Case 2 is the equiangular spiral, which is the spiral par excellence. This has great historical significance as in Proposition 9 of the Principia Book 1, Newton proves that if a body moves along an equiangular spiral, under the action of a central force, that force must be as the inverse of the cube of the radius (even before his proof, in Proposition 11, that motion in an ellipse directed to a focus requires an inverse-square force).

It has to be admitted that not all the curves conform to the usual definition of a spiral. For example, when the inverse-cube force is centrifugal (directed outwards), so that μ < 0, the curve does not even rotate once about the centre. This is represented by case 5, the first of the polar equations shown above, with k > 1 in this case.

Earnshaw clearly describes Cotes's 5 cases and unnecessarily adds a 6th, which is when the force is centrifugal (repulsive). As noted above, Cotes's included this with case 5.

The mistaken view that there are only 3 Cotes's spirals appears to have originated with Whittaker's "A Treatise on the Analytical Dynamics of Particles and Rigid Bodies", first published in 1904.

Whittaker's "reciprocal spiral" does have a footnote, which refers to Cotes's "Harmonia Mensurarum" and Newton's Proposition 9. However, it is misleading, since the spiral of Proposition 9 is the equiangular spiral, which he does not recognise as a Cotes's spiral at all.

Unfortunately, subsequent authors have followed Whittaker's lead without taking the trouble to verify its accuracy.

See also

References

1. ^{{cite book |url=https://books.google.com/books?id=mms6MXH9CuoC&pg=PA34 |title=The sheer joy of celestial mechanics |author=Nathaniel Grossman |publisher=Springer |year=1996 |isbn=978-0-8176-3832-0 |page=34}}
2. ^{{Cite book |url=https://archive.org/details/treatisanalytdyn00whitrich |title=A treatise on the analytical dynamics of particles and rigid bodies; with an introduction to the problem of three bodies |last=Whittaker |first=Edmund Taylor |date= |publisher= |year=1917 |isbn= |edition=Second |location=Cambridge University Press |pages=83 |language=English}}
3. ^{{Cite book |url=https://archive.org/details/dynamicsoranele00earngoog |title=Dynamics, Or an Elementary Treatise On Motion; With a Great Variety of Examples Illustrative of the General Principles and Formulae: To Which Is Added a Short Treatise On Attractions |last=Earnshaw |first=Samuel |date= |publisher=Printed by W. Metcalfe, for J. & J. J. Deighton |year=1832 |isbn= |location=Cambridge |pages=47 |language=English}}

Classical mechanics

History

See also

References

Bibliography

External links