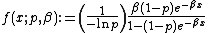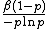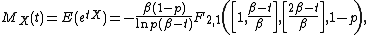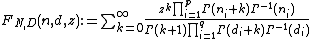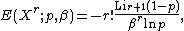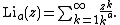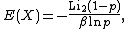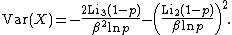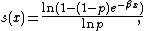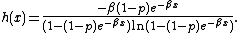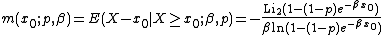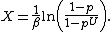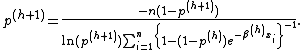- Introduction
- Properties of the distribution Distribution Moments The survival, hazard and mean residual life functions Random number generation
- Estimation of the parameters
- Related distributions
- References
| name = Exponential-Logarithmic distribution (EL)
| type = continuous
| pdf_image =
| cdf_image =
| notation =
| parameters =
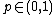
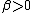
| support =
| pdf =
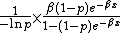
| cdf =
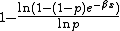
| mean =
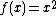
| median =
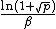
| mode = 0
| variance =
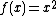
| skewness =
| kurtosis =
| entropy =
| mgf =
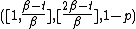
| cf =
| pgf =
| fisher =
}}
In probability theory and statistics, the Exponential-Logarithmic (EL) distribution is a family of lifetime distributions with
decreasing failure rate, defined on the interval [0, ∞). This distribution is parameterized by two parameters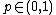 
and .
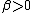Introduction
The study of lengths of the lives of organisms, devices, materials, etc., is of major importance in the biological and engineering sciences. In general, the lifetime of a device is expected to exhibit decreasing failure rate (DFR) when its behavior over time is characterized by 'work-hardening' (in engineering terms) or 'immunity' (in biological terms).
The exponential-logarithmic model, together with its various properties, are studied by Tahmasbi and Rezaei (2008)[1]
This model is obtained under the concept of population heterogeneity (through the process of
compounding).
Properties of the distribution
Distribution
The probability density function (pdf) of the EL distribution is given by Tahmasbi and Rezaei (2008)[1]
where 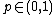and 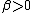. This function is strictly decreasing in 
and tends to zero as 
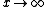. The EL distribution has its modal value of the density at x=0, given by
The EL reduces to the exponential distribution with rate parameter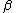 
, as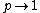 
.
The cumulative distribution function is given by
and hence, the median is given by
.
Moments
The moment generating function of 
can be determined from the pdf by direct integration and is given by
where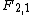 
is a hypergeometric function. This function is also known as Barnes's extended hypergeometric function. The definition of 
is
where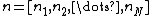 
and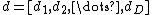 
.
The moments of can be derived from 
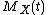. For
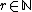, the raw moments are given by
where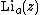 
is the polylogarithm function which is defined as
follows:[2]
Hence the mean and variance of the EL distribution
are given, respectively, by
The survival, hazard and mean residual life functions
The survival function (also known as the reliability
function) and hazard function (also known as the failure rate
function) of the EL distribution are given, respectively, by
The mean residual lifetime of the EL distribution is given by
where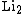 
is the dilogarithm function
Random number generation
Let U be a random variate from the standard uniform distribution.
Then the following transformation of U has the EL distribution with
parameters p and β:
Estimation of the parameters
To estimate the parameters, the EM algorithm is used. This method is discussed by Tahmasbi and Rezaei (2008).[1] The EM iteration is given by
Related distributions
The EL distribution has been generalized to form the Weibull-logarithmic distribution.[3]
If X is defined to be the random variable which is the minimum of N independent realisations from an exponential distribution with rate parameter β, and if N is a realisation from a logarithmic distribution (where the parameter p in the usual parameterisation is replaced by {{nowrap|1=(1 − p)}}), then X has the exponential-logarithmic distribution in the parameterisation used above.
References
2. ^Lewin, L. (1981) Polylogarithms and Associated Functions, NorthHolland, Amsterdam.
3. ^Ciumara, Roxana; Preda, Vasile (2009) "The Weibull-logarithmic distribution in lifetime analysis and its properties". In: L. Sakalauskas, C. Skiadas andE. K. Zavadskas (Eds.) Applied Stochastic Models and Data Analysis, The XIII International Conference, Selected papers. Vilnius, 2009 {{ISBN|978-9955-28-463-5}}
- World Open Solar Energy Journal
- World Open Sol Energy J
- World Open Sol. Energy J.
- World Open Solid State Chemistry Journal
- World Open Solid State Chem. J.
- World Open Solid State Chem J
- World Open Solid State Physics Journal
- World Open Solid State Phys J
- World Open Solid State Phys. J.
- World Open Soybeans and Sorghum Research Journal
- World Open Soybeans & Sorghum Research Journal
- World Open Soybeans Sorghum Res. J.
- World Open Soybeans Sorghum Res J
- World Open Spectral Analysis Review Journal
- World Open Spectr Anal Rev J
- World Open Spectr. Anal. Rev. J.
- World Open Spectrosc J
- World Open Spectrosc. J.
- World Open Spectroscopy Journal
- World Open Spinal Cord J.
- World Open Spinal Cord J
- World Open Spinal Cord Journal
- World Open Sports Medicine Journal
- World Open Sports Med. J.
- World Open Sports Med J