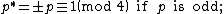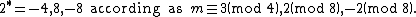“Genus field”的意思、由来-开放百科全书

词条

Genus field

释义

See also
References

In algebraic number theory, the genus field G of an algebraic number field K is the maximal abelian extension of K which is obtained by composing an absolutely abelian field with K and which is unramified at all finite primes of K. The genus number of K is the degree [G:K] and the genus group is the Galois group of G over K.

If K is itself absolutely abelian, the genus field may be described as the maximal absolutely abelian extension of K unramified at all finite primes: this definition was used by Leopoldt and Hasse.

If K=Q({{radic|m}}) (m squarefree) is a quadratic field of discriminant D, the genus field of K is a composite of quadratic fields. Let p_i run over the prime factors of D. For each such prime p, define p^∗ as follows:

Then the genus field is the composite

References

{{cite book | last=Ishida | first=Makoto | title=The genus fields of algebraic number fields | series=Lecture Notes in Mathematics | volume=555 | publisher=Springer-Verlag | year=1976 | isbn=3-540-08000-7 | zbl=0353.12001 }}
{{cite book | first=Gerald | last=Janusz | title=Algebraic Number Fields | year=1973 | publisher=Academic Press | isbn=0-12-380250-4 | series=Pure and Applied Mathematics | volume=55 | zbl=0307.12001 }}
{{cite book | last=Lemmermeyer | first= Franz | title=Reciprocity laws. From Euler to Eisenstein | series= Springer Monographs in Mathematics | publisher=Springer-Verlag | location=Berlin | year= 2000 | isbn= 3-540-66957-4 | url=https://books.google.com/books?id=EwjpPeK6GpEC | mr=1761696 | zbl=0949.11002 }}

1 : Class field theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

See also

References