“Hyperfinite set”的意思、由来-开放百科全书

In non-standard analysis, a branch of mathematics, a hyperfinite set or *-finite set is a type of internal set. An internal set H of internal cardinality g ∈ *N (the hypernaturals) is hyperfinite if and only if there exists an internal bijection between G = {1,2,3,...,g} and H.^[1]^[2] Hyperfinite sets share the properties of finite sets: A hyperfinite set has minimal and maximal elements, and a hyperfinite union of a hyperfinite collection of hyperfinite sets may be derived. The sum of the elements of any hyperfinite subset of *R always exists, leading to the possibility of well-defined integration.^[2]

Hyperfinite sets can be used to approximate other sets. If a hyperfinite set approximates an interval, it is called a near interval with respect to that interval. Consider a hyperfinite set

with a hypernatural n. K is a near interval for [a,b] if k₁ = a and k_n = b, and if the difference between successive elements of K is infinitesimal. Phrased otherwise, the requirement is that for every r ∈ [a,b] there is a k_i ∈ K such that k_i ≈ r. This, for example, allows for an approximation to the unit circle, considered as the set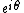

for θ in the interval [0,2π].^[2]

In general, subsets of hyperfinite sets are not hyperfinite, often because they do not contain the extreme elements of the parent set.^[3]

Ultrapower construction

In terms of the ultrapower construction, the hyperreal line *R is defined as the collection of equivalence classes of sequences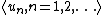

of real numbers u_n. Namely, the equivalence class defines a hyperreal, denoted

in Goldblatt's notation. Similarly, an arbitrary hyperfinite set in *R is of the form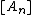

, and is defined by a sequence

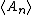of finite sets

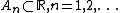^[4]

Notes

1. ^{{cite book|title=Optimization and nonstandard analysis|author=J. E. Rubio|publisher=Marcel Dekker|year=1994|isbn=0-8247-9281-5|page=110}}
2. ^¹²{{cite book|title=Truth, possibility, and probability: new logical foundations of probability and statistical inference|author=R. Chuaqui|authorlink= Rolando Chuaqui|publisher=Elsevier|year=1991|isbn=0-444-88840-3|pages=182–3}}
3. ^{{cite book|title=Calculus of variations and partial differential equations: topics on geometrical evolution problems and degree theory|author=L. Ambrosio|publisher=Springer|year=2000|isbn=3-540-64803-8|page=203|display-authors=etal}}
4. ^{{cite book|author=R. Goldblatt|year=1998|title=Lectures on the hyperreals. An introduction to nonstandard analysis|page=188|publisher=Springer|isbn=0-387-98464-X}}

Ultrapower construction

Notes

External links