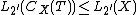“L-balance theorem”的意思、由来-开放百科全书

词条

L-balance theorem

释义

Statement
References

In mathematical finite group theory, the L-balance theorem was proved by {{harvtxt|Gorenstein|Walter|1975}}.

The letter L stands for the layer of a group, and "balance" refers to the property discussed below.

Statement

The L-balance theorem of Gorenstein and Walter states that if X is a finite group and T a 2-subgroup of X then

Here L_2′(X) stands for the 2-layer of a group X, which is the product of all the 2-components of the group, the minimal subnormal subgroups of X mapping onto components of X/O(X).

A consequence is that if a and b are commuting involutions of a group G then

This is the property called L-balance.

More generally similar results are true if the prime 2 is replaced by a prime p, and in this case the condition is called L_p-balance, but the proof of this requires the classification of finite simple groups (more precisely the Schreier conjecture).

References

{{Citation | last1=Gorenstein | first1=D. | author1-link=Daniel Gorenstein | last2=Walter | first2=John H. | title=Balance and generation in finite groups | doi=10.1016/0021-8693(75)90123-4 | mr=0357583 | year=1975 | journal=Journal of Algebra | issn=0021-8693 | volume=33 | pages=224–287}}

2 : Finite groups|Theorems in group theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。