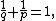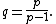“List of Banach spaces”的意思、由来-开放百科全书

词条

List of Banach spaces

释义

Classical Banach spaces
Banach spaces in other areas of analysis
Banach spaces serving as counterexamples
Notes
References

In the mathematical field of functional analysis, Banach spaces are among the most important objects of study. In other areas of mathematical analysis, most spaces which arise in practice turn out to be Banach spaces as well.

Classical Banach spaces

According to {{harvtxt|Diestel|1984|loc=Chapter VII}}, the classical Banach spaces are those defined by {{harvtxt|Dunford|Schwartz|1958}}, which is the source for the following table.

Here K denotes the field of real numbers or complex numbers and I is a closed and bounded interval [a,b]. The number p is a real number with {{nowrap|1 < p < ∞}}, and q is its Hölder conjugate (also with {{nowrap|1 < q < ∞}}), so that the next equation holds:

and thus

The symbol Σ denotes a σ-algebra of sets, and Ξ denotes just an algebra of sets (for spaces only requiring finite additivity, such as the ba space). The symbol μ denotes a positive measure: that is, a real-valued positive set function defined on a σ-algebra which is countably additive.

Dual space	Reflexive	weakly complete	Norm	Notes
Classical Banach spaces
Kⁿ	Kⁿ	{{yes}}	{{yes}}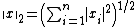
ℓⁿ_p	ℓⁿ_q	{{yes	{{yes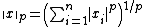
ℓⁿ_∞	ℓⁿ₁	{{yes}}	{{yes}}
ℓ_p	ℓ_q	{{yes	{{yes		1 < p < ∞
ℓ₁	ℓ_∞	{{no	{{yes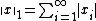
ℓ_∞	ba	{{no	{{no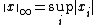
c	ℓ₁	{{no}}	{{no}}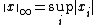
c₀	ℓ₁	{{no}}	{{no}}		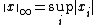Isomorphic but not isometric to c.
bv	ℓ₁ + K	{{no}}	{{yes}}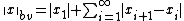
bv₀	ℓ₁	{{no}}	{{yes}}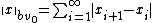
bs	ba	{{no}}	{{no}}		Isometrically isomorphic to ℓ_∞.
cs	ℓ₁	{{no}}	{{no}}		Isometrically isomorphic to c.
B(X, Ξ)	ba(Ξ)	{{no	{{no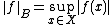
C(X)	rca(X)	{{no}}	{{no}}		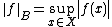X is a compact Hausdorff space.
ba(Ξ)	?	{{no}}	{{yes}}	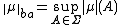(variation of a measure)
ca(Σ)	?	{{no}}	{{yes}}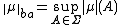
rca(Σ)	?	{{no}}	{{yes}}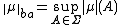
L^p(μ)	L^q(μ)	{{yes}}	{{yes}}		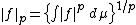1 < p < ∞
L¹(μ)	L^∞(μ)	{{no}}	?		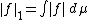If the measure μ on S is sigma-finite
L^∞(μ)		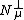{{no}}	?		where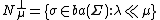
BV(I)	?	{{no}}	{{yes}}		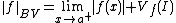V_f(I) is the total variation of f.
NBV(I)	?	{{no}}	{{yes}}		NBV(I) consists of BV functions such that .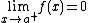
AC(I)	K+L^∞(I)	{{no}}	{{yes}}		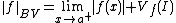Isomorphic to the Sobolev space W^1,1(I).
Cⁿ[a,b]	rca([a,b])	{{no}}	{{no}}		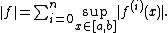Isomorphic to Rⁿ ⊕ C([a,b]), essentially by Taylor's theorem.

Banach spaces in other areas of analysis

The Asplund spaces
The Hardy spaces
The space BMO of functions of bounded mean oscillation
The space of functions of bounded variation
Sobolev spaces
The Birnbaum–Orlicz spaces L_A(μ).
Hölder spaces C^k,α(Ω).
Lorentz space

Banach spaces serving as counterexamples

James' space, a Banach space that has a Schauder basis, but has no unconditional Schauder Basis. Also, James' space is isometrically isomorphic to its double dual, but fails to be reflexive.
Tsirelson space, a reflexive Banach space in which neither ℓ^p nor c₀ can be embedded.
W.T. Gowers construction of a space X that is isomorphic to 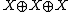but not 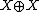serves as a counterexample for weakening the premises of the Schroeder–Bernstein theorem ^[1]

Notes

1. ^W.T. Gowers, "A solution to the Schroeder–Bernstein problem for Banach spaces", Bulletin of the London Mathematical Society, 28 (1996) pp. 297–304.

References

{{citation|title=Sequences and series in Banach spaces|first=Joseph|last=Diestel|publisher=Springer-Verlag|year=1984|isbn=0-387-90859-5}}.
{{citation|first1=N.|last1=Dunford|first2=J.T.|last2=Schwartz|title=Linear operators, Part I|publisher=Wiley-Interscience|year=1958}}.

1 : Banach spaces

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。