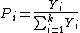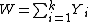- The theorem Corollary
- References
In statistics, Lukacs's proportion-sum independence theorem is a result that is used when studying proportions, in particular the Dirichlet distribution. It is named for Eugene Lukacs.[1]
The theorem
If Y1 and Y2 are non-degenerate, independent random variables, then the random variables
are independently distributed if and only if both Y1 and Y2 have gamma distributions with the same scale parameter.
Corollary
Suppose Y i, i = 1, ..., k be non-degenerate, independent, positive random variables. Then each of k − 1 random variables
is independent of
if and only if all the Y i have gamma distributions with the same scale parameter.[2]
References
1. ^{{Cite journal|doi= 10.1214/aoms/1177728549|author= Lukacs, Eugene|title=A characterization of the gamma distribution|journal=Annals of Mathematical Statistics|volume=26|year=1955|pages=319–324}}
2. ^{{cite journal| last=Mosimann| first=James E.| title=On the compound multinomial distribution, the multivariate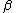 distribution, and correlation among proportions| journal=Biometrika| year=1962| volume=49| issue=1 and 2|pages=65–82|jstor=2333468| doi=10.1093/biomet/49.1-2.65}}
distribution, and correlation among proportions| journal=Biometrika| year=1962| volume=49| issue=1 and 2|pages=65–82|jstor=2333468| doi=10.1093/biomet/49.1-2.65}}
2. ^{{cite journal| last=Mosimann| first=James E.| title=On the compound multinomial distribution, the multivariate
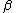 distribution, and correlation among proportions| journal=Biometrika| year=1962| volume=49| issue=1 and 2|pages=65–82|jstor=2333468| doi=10.1093/biomet/49.1-2.65}}
distribution, and correlation among proportions| journal=Biometrika| year=1962| volume=49| issue=1 and 2|pages=65–82|jstor=2333468| doi=10.1093/biomet/49.1-2.65}}- {{cite book|last1=Ng| first1=W. N.|last2=Tian| first2=G-L| last3=Tang| first3=M-L| title=Dirichlet and Related Distributions| publisher=John Wiley & Sons, Ltd.| year=2011|isbn=978-0-470-68819-9}} page 64. [https://books.google.com/books?id=k8GS868oyo4C&pg=PT81&dq#v=onepage&q&f=false Lukacs's proportion-sum independence theorem and the corollary] with a proof.
2 : Probability theorems|Characterization of probability distributions
- Rabbit Test (Ugly Betty)
- Rabbit Track Line
- Rabbit Transit
- Rabbit Transit (cartoon)
- Rabbit Transit (disambiguation)
- Rabbit transit (game)
- Rabbit Transit (game)
- Rabbit Transit (York)
- Rabbit Transit (York, PA)
- Rabbitt Trax
- Rabbit valley, colorado
- Rabbit Valley (Colorado)
- RabbitVCS
- Rabbitville
- Rabbitville, Indiana
- Rabbit virus
- "Rabbit" Weller
- Rabbit Weller
- Rabbit (Winnie the Pooh)
- Rabbit Without Ears
- Rabbit Without Ears 2
- Rabbit à la Berlin
- Rabbit (卯)
- Rabbi Uri Feibusch Hart
- Rabbi Uri Feibush Hart