- Definition
- Examples
- Properties
- See also
- References
- Further reading Historical Modern
- External links
In mathematics, the Lebesgue covering dimension or topological dimension of a topological space is one of several different ways of defining the dimension of the space in a
topologically invariant way.
Definition
The first formal definition of covering dimension was given by Eduard Čech, based on an earlier result of Henri Lebesgue.[1]
A modern definition is as follows. An open cover of a topological space X is a family of open sets whose union contains X. The ply or order of a cover is the smallest number n (if it exists) such that each point of the space belongs to at most n sets in the cover. A refinement of a cover C is another cover, each of whose sets is a subset of a set in C; its ply may be smaller than, or possibly larger than, the ply of C.
The covering dimension of a topological space X is defined to be the minimum value of n, such that every open cover C of X has an open refinement with ply n + 1 or below. If no such minimal n exists, the space is said to be of infinite covering dimension.
As a special case, a topological space is zero-dimensional with respect to the covering dimension if every open cover of the space has a refinement consisting of disjoint open sets so that any point in the space is contained in exactly one open set of this refinement.
Examples
Any given open cover of the unit circle will have a refinement consisting of a collection of open arcs. The circle has dimension one, by this definition, because any such cover can be further refined to the stage where a given point x of the circle is contained in at most two open arcs. That is, whatever collection of arcs we begin with, some can be discarded or shrunk, such that the remainder still covers the circle but with simple overlaps.
Similarly, any open cover of the unit disk in the two-dimensional plane can be refined so that any point of the disk is contained in no more than three open sets, while two are in general not sufficient. The covering dimension of the disk is thus two.
More generally, the n-dimensional Euclidean space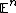 
has covering dimension n.
A non-technical illustration of these examples is given below.
Properties
- Homeomorphic spaces have the same covering dimension. That is, the covering dimension is a topological invariant.
- The Lebesgue covering dimension coincides with the affine dimension of a finite simplicial complex; this is the Lebesgue covering theorem.
- The covering dimension of a normal space is less than or equal to the large inductive dimension.
- Covering dimension of a normal space X is
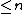if and only if for any closed subset A of X, if 
is continuous, then there is an extension of 
to 
. Here, 
is the n dimensional sphere. - (Ostrand's theorem on colored dimension.) A normal space
satisfies the inequality 
if and only if for every locally finite open cover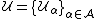 
of the space there exists an open cover 
of the space which can be represented as the union of 
families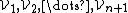 
, where 
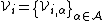, such that each 
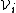contains disjoint sets and 
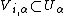for each 
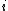and 
. - The covering dimension of a paracompact Hausdorff space is greater or equal to its cohomological dimension (in the sense of sheaves),[2] that is one has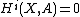
for every sheaf 
of abelian groups on and every 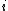larger than the covering dimension of .
See also
- Carathéodory's extension theorem
- Geometric set cover problem
- Dimension theory
- Metacompact space
- Point-finite collection
References
2. ^Godement 1973, II.5.12, p. 236
- {{Citation | last1=Godement | first1=Roger | author1-link = Roger Godement | title=Topologie algébrique et théorie des faisceaux | publisher=Hermann | location=Paris | mr=0345092 | year=1973}}
- {{cite book |ref= harv | last=Munkres |first=James R. |authorlink=James Munkres|title=Topology |year=2000 |edition=2nd |publisher=Prentice-Hall |isbn=0-13-181629-2}}
Further reading
Historical
- Karl Menger, General Spaces and Cartesian Spaces, (1926) Communications to the Amsterdam Academy of Sciences. English translation reprinted in Classics on Fractals, Gerald A.Edgar, editor, Addison-Wesley (1993) {{isbn|0-201-58701-7}}
- Karl Menger, Dimensionstheorie, (1928) B.G Teubner Publishers, Leipzig.
- A. R. Pears, Dimension Theory of General Spaces, (1975) Cambridge University Press. {{isbn|0-521-20515-8}}
Modern
- V.V. Fedorchuk, The Fundamentals of Dimension Theory, appearing in Encyclopaedia of Mathematical Sciences, Volume 17, General Topology I, (1993) A. V. Arkhangel'skii and L. S. Pontryagin (Eds.), Springer-Verlag, Berlin {{isbn|3-540-18178-4}}.
External links
- {{springer|title=Lebesgue dimension|id=p/l057830}}
- Hector Manzanilla
- Hector Marinaro, Sr.
- Hector Martin
- Hector Martinez
- Hector Martinez (baseball)
- Hector Martinez Munoz
- Hector-Martin Lefuel
- Hector Maseda Gutierrez
- Hector McIvor
- Hector McLean
- Hector McLeod Sam
- Hector Medrano
- Hector Melesio Cuen Ojeda
- Hector melesio cuen ojeda
- Hector Memorial Medal
- Hector Mendez (rugby)
- Hector Mercado
- Hector Mine
- Hector Mine earthquake
- Hector M. Laffitte
- Hector Monro (disambiguation)
- Hector Montenegro
- Hector Morales (footballer)
- Hector Moreno (footballer)
- Hector Morison