“Ordinal definable set”的意思、由来-开放百科全书

词条

Ordinal definable set

释义

References

In mathematical set theory, a set S is said to be ordinal definable if, informally, it can be defined in terms of a finite number of ordinals by a first order formula. Ordinal definable sets were introduced by {{harvtxt|Gödel|1965}}.

A drawback to this informal definition is that requires quantification over all first order formulas, which cannot be formalized in the language of set theory. However there is a different way of stating the definition that can be so formalized. In this approach, a set S is formally defined to be ordinal definable if there is some collection of ordinals α₁...α_n such that 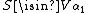and can be defined as an element of 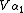by a first-order formula φ taking α₂...α_n as parameters. Here 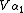denotes the set indexed by the ordinal α₁ in the von Neumann hierarchy of sets. In other words, S is the unique object such that φ(S, α₂...α_n) holds with its quantifiers ranging over .

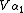The class of all ordinal definable sets is denoted OD; it is not necessarily transitive, and need not be a model of ZFC because it might not satisfy the axiom of extensionality. A set is hereditarily ordinal definable if it is ordinal definable and all elements of its transitive closure are ordinal definable. The class of hereditarily ordinal definable sets is denoted by HOD, and is a transitive model of ZFC, with a definable well ordering. It is consistent with the axioms of set theory that all sets are ordinal definable, and so hereditarily ordinal definable. The assertion that this situation holds is referred to as V = OD or V = HOD. It follows from V = L, and is equivalent to the existence of a (definable) well-ordering of the universe. Note however that the formula expressing V = HOD need not hold true within HOD, as it is not absolute for models of set theory: within HOD, the interpretation of the formula for HOD may yield an even smaller inner model.

HOD has been found to be useful in that it is an inner model that can accommodate essentially all known large cardinals. This is in contrast with the situation for core models, as core models have not yet been constructed that can accommodate supercompact cardinals, for example.

References

{{Citation | last1=Gödel | first1=Kurt | editor1-last=Davis | editor1-first=Martin | editor1-link=Martin Davis | title=The undecidable. Basic papers on undecidable propositions, unsolvable problems and computable functions | origyear=1946 | publisher=Raven Press, Hewlett, N.Y. |mr=0189996 | year=1965 | chapter=Remarks before the Princeton Bicentennial Conference on Problems in Mathematics | pages=84–88|isbn=978-0-486-43228-1}}
{{Citation | last1=Kunen | first1=Kenneth | author1-link=Kenneth Kunen | title=Set theory: An introduction to independence proofs | publisher=Elsevier | isbn=978-0-444-86839-8 | year=1980}}

1 : Set theory

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。