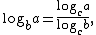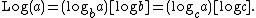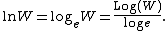“Logarithmic scale”的意思、由来-开放百科全书

词条

Logarithmic scale

释义

Common usages
Log–log plots Semi-logarithmic plots
Logarithmic units
Examples Units of information Units of level or level difference Units of frequency interval Motivation
See also
Scale Applications
References
Further reading
External links

A logarithmic scale is a nonlinear scale used when there is a large range of quantities. Common uses include earthquake strength, sound loudness, light intensity, and pH of solutions.

It is based on orders of magnitude, rather than a standard linear scale, so the value represented by each equidistant mark on the scale is the value at the previous mark multiplied by a constant.

Logarithmic scales are also used in slide rules for multiplying or dividing numbers by adding or subtracting lengths on the scales.

Common usages

The following are examples of commonly used logarithmic scales, where a larger quantity results in a higher value:

Richter magnitude scale and moment magnitude scale (MMS) for strength of earthquakes and movement in the earth
sound level, with units bel and decibel
neper for amplitude, field and power quantities
frequency level, with units cent, minor second, major second, and octave for the relative pitch of notes in music
logit for odds in statistics
Palermo Technical Impact Hazard Scale
logarithmic timeline
counting f-stops for ratios of photographic exposure
the rule of 'nines' used for rating low probabilities
entropy in thermodynamics
information in information theory
particle-size-distribution curves of soil

The following are examples of commonly used logarithmic scales, where a larger quantity results in a lower (or negative) value:

pH for acidity
stellar magnitude scale for brightness of stars
Krumbein scale for particle size in geology
absorbance of light by transparent samples

Some of our senses operate in a logarithmic fashion (Weber–Fechner law), which makes logarithmic scales for these input quantities especially appropriate. In particular our sense of hearing perceives equal ratios of frequencies as equal differences in pitch. In addition, studies of young children in an isolated tribe have shown logarithmic scales to be the most natural display of numbers in some cultures.^[1] It can also be used for geographical purposes like for measuring the speed of earthquakes.

==Graphic representation==

The top left graph is linear in the X and Y axis, and the Y-axis ranges from 0 to 10. A base-10 log scale is used for the Y axis of the bottom left graph, and the Y axis ranges from 0.1 to 1,000.

The top right graph uses a log-10 scale for just the X axis, and the bottom right graph uses a log-10 scale for both the X axis and the Y axis.

Presentation of data on a logarithmic scale can be helpful when the data:

covers a large range of values, since the use of the logarithms of the values rather than the actual values reduces a wide range to a more manageable size;
may contain exponential laws or power laws, since these will show up as straight lines.

A slide rule has logarithmic scales, and nomograms often employ logarithmic scales. The geometric mean of two numbers is midway between the numbers. Before the advent of computer graphics, logarithmic graph paper was a commonly used scientific tool.

Log–log plots

If both the vertical and horizontal axes of a plot are scaled logarithmically, the plot is referred to as a log–log plot.

Semi-logarithmic plots

If only the ordinate or abscissa is scaled logarithmically, the plot is referred to as a semi-logarithmic plot.

Logarithmic units

A logarithmic unit is a unit that can be used to express a quantity (physical or mathematical) on a logarithmic scale, that is, as being proportional to the value of a logarithm function applied to the ratio of the quantity and a reference quantity of the same type. The choice of unit generally indicates the type of quantity and the base of the logarithm.

Examples

Examples of logarithmic units include units of data storage capacity (bit, byte), of information and information entropy (nat, shannon, ban), signal level (decibel, bel, neper). Logarithmic frequency quantities are used in electronics (decade, octave) and for music pitch intervals (octave, semitone, cent, etc.). Other logarithmic scale units include the Richter magnitude scale point.

Units of information

bit, byte
hartley
nat
shannon

Units of level or level difference

bel, decibel
neper

Units of frequency interval

decade, decidecade, savart
octave, tone, semitone, cent

Motivation

The motivation behind the concept of logarithmic units is that defining a quantity on a logarithmic scale in terms of a logarithm to a specific base amounts to making a (totally arbitrary) choice of a unit of measurement for that quantity, one that corresponds to the specific (and equally arbitrary) logarithm base that was selected. Due to the identity

the logarithms of any given number a to two different bases (here b and c) differ only by the constant factor log_c b. This constant factor can be considered to represent the conversion factor for converting a numerical representation of the pure (indefinite) logarithmic quantity Log(a) from one arbitrary unit of measurement (the [log c] unit) to another (the [log b] unit), since

For example, Boltzmann's standard definition of entropy S = k ln W (where W is the number of ways of arranging a system and k is Boltzmann's constant) can also be written more simply as just S = Log(W), where "Log" here denotes the indefinite logarithm, and we let k = [log e]; that is, we identify the physical entropy unit k with the mathematical unit [log e]. This identity works because

Thus, we can interpret Boltzmann's constant as being simply the expression (in terms of more standard physical units) of the abstract logarithmic unit [log e] that is needed to convert the dimensionless pure-number quantity ln W (which uses an arbitrary choice of base, namely e) to the more fundamental pure logarithmic quantity Log(W), which implies no particular choice of base, and thus no particular choice of physical unit for measuring entropy.

References

1. ^{{cite web|url=https://www.sciencedaily.com/releases/2008/05/080529141344.htm|title=Slide Rule Sense: Amazonian Indigenous Culture Demonstrates Universal Mapping Of Number Onto Space|date=2008-05-30|publisher=ScienceDaily|accessdate=2008-05-31}}

External links

{{cite web|url=https://www.gnu.org/software/emacs/manual/html_node/calc/Logarithmic-Units.html |title=GNU Emacs Calc Manual: Logarithmic Units |website=Gnu.org |date= |accessdate=2016-11-23}}
[https://sites.google.com/site/nonnewtoniancalculus/ Non-Newtonian calculus website]

2 : Logarithmic scales of measurement|Non-Newtonian calculus

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。