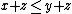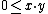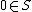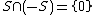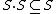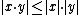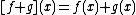- Properties
- f-rings Example Properties
- Formally verified results for commutative ordered rings
- References
- Further reading
- External links
In abstract algebra, a partially ordered ring is a ring (A, +, · ), together with a compatible partial order, i.e. a partial order 
on the underlying set A that is compatible with the ring operations in the sense that it satisfies:
implies
and
and
imply that
for all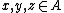 
.[1] Various extensions of this definition exist that constrain the ring, the partial order, or both. For example, an Archimedean partially ordered ring is a partially ordered ring 
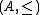where 
's partially ordered additive group is Archimedean.[2]
An ordered ring, also called a totally ordered ring, is a partially ordered ring 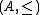where 
is additionally a total order.[1][2]
An l-ring, or lattice-ordered ring, is a partially ordered ring 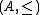where is additionally a lattice order.
Properties
The additive group of a partially ordered ring is always a partially ordered group.
The set of non-negative elements of a partially ordered ring (the set of elements x for which 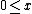, also called the positive cone of the ring) is closed under addition and multiplication, i.e., if P is the set of non-negative elements of a partially ordered ring, then 
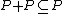, and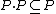 
. Furthermore, 
.
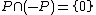The mapping of the compatible partial order on a ring A to the set of its non-negative elements is one-to-one;[1] that is, the compatible partial order uniquely determines the set of non-negative elements, and a set of elements uniquely determines the compatible partial order if one exists.
If S is a subset of a ring A, and:
then the relation where iff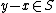 
defines a compatible partial order on A (ie. 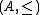is a partially ordered ring).[2]
In any l-ring, the absolute value 
of an element x can be defined to be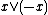 
, where 
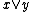denotes the maximal element. For any x and y,
holds.[3]
f-rings
An f-ring, or Pierce–Birkhoff ring, is a lattice-ordered ring 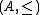in which 
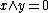[4] and 
imply that 
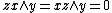for all 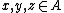. They were first introduced by Garrett Birkhoff and Richard S. Pierce in 1956, in a paper titled "Lattice-ordered rings", in an attempt to restrict the class of l-rings so as to eliminate a number of pathological examples. For example, Birkhoff and Pierce demonstrated an l-ring with 1 in which 1 is negative, even though being a square.[2] The additional hypothesis required of f-rings eliminates this possibility.
Example
Let X be a Hausdorff space, and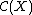 
be the space of all continuous, real-valued functions on X. 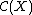is an Archimedean f-ring with 1 under the following point-wise operations:
[2]
From an algebraic point of view the rings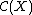
are fairly rigid. For example, localisations, residue rings or limits of
rings of the form 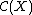are not of this form in general.
A much more flexible class of f-rings containing all rings of continuous functions
and resembling many of the properties of these rings, is the class of real closed rings.
Properties
A direct product of f-rings is an f-ring, an l-subring of an f-ring is an f-ring, and an l-homomorphic image of an f-ring is an f-ring.[3]
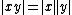in an f-ring.[3]The category Arf consists of the Archimedean f-rings with 1 and the l-homomorphisms that preserve the identity.[5]
Every ordered ring is an f-ring, so every subdirect union of ordered rings is also an f-ring. Assuming the axiom of choice, a theorem of Birkhoff shows the converse, and that an l-ring is an f-ring if and only if it is l-isomorphic to a subdirect union of ordered rings.[2] Some mathematicians take this to be the definition of an f-ring.[3]
Formally verified results for commutative ordered rings
IsarMathLib, a library for the Isabelle theorem prover, has formal verifications of a few fundamental results on commutative ordered rings. The results are proved in the ring1 context.[6]Suppose 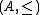is a commutative ordered ring, and 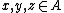. Then:
| by | |
|---|---|
| The additive group of A is an ordered group | OrdRing_ZF_1_L4 |
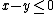iff  | OrdRing_ZF_1_L7 |
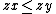and imply and  | OrdRing_ZF_1_L9 |
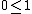 | ordring_one_is_nonneg |
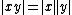| | OrdRing_ZF_2_L5 |
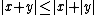 | ord_ring_triangle_ineq |
| x is either in the positive set, equal to 0, or in minus the positive set. | OrdRing_ZF_3_L2 |
| The set of positive elements of 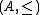is closed under multiplication iff A has no zero divisors. | OrdRing_ZF_3_L3 |
If A is non-trivial (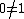 ), then it is infinite. | ord_ring_infinite |
References
2. ^1 2 3 4 5 {{cite journal| last = Johnson | first = D. G. |date=December 1960 | title = A structure theory for a class of lattice-ordered rings | journal = Acta Mathematica | volume = 104 | issue = 3–4 | pages = 163–215 | doi = 10.1007/BF02546389}}
3. ^1 2 3 {{cite book| last = Henriksen | first = Melvin | authorlink = Melvin Henriksen | chapter = A survey of f-rings and some of their generalizations | pages = 1–26 | title = Ordered Algebraic Structures: Proceedings of the Curaçao Conference Sponsored by the Caribbean Mathematics Foundation, June 23–30, 1995 | year = 1997 | editor = W. Charles Holland and Jorge Martinez | isbn = 0-7923-4377-8 | publisher = Kluwer Academic Publishers | location = the Netherlands}}
4. ^
denotes infimum.5. ^{{cite journal| last = Hager | first = Anthony W. |author2=Jorge Martinez | year = 2002 | title = Functorial rings of quotients—III: The maximum in Archimedean f-rings | journal = Journal of Pure and Applied Algebra | volume = 169 | pages = 51–69| doi = 10.1016/S0022-4049(01)00060-3}}
6. ^{{cite web| url = http://www.nongnu.org/isarmathlib/IsarMathLib/document.pdf | title = IsarMathLib | accessdate = 2009-03-31}}
Further reading
- {{cite journal| last = Birkhoff | first = G. |author2=R. Pierce | year = 1956 | title = Lattice-ordered rings | journal = Anais da Academia Brasileira de Ciências | volume = 28 | pages = 41–69}}
- Gillman, Leonard; Jerison, Meyer Rings of continuous functions. Reprint of the 1960 edition. Graduate Texts in Mathematics, No. 43. Springer-Verlag, New York-Heidelberg, 1976. xiii+300 pp
External links
- {{cite web| title = Ordered Ring, Partially Ordered Ring | publisher = Encyclopedia of Mathematics | url = http://eom.springer.de/O/o070140.htm | accessdate = 2009-04-03}}
- {{cite web| title = Partially Ordered Ring | publisher = PlanetMath | url = http://planetmath.org/PartiallyOrderedRing | accessdate = 2018-04-14}}