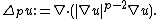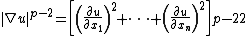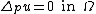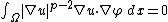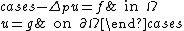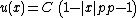“P-Laplacian”的意思、由来-开放百科全书

词条

P-Laplacian

释义

Energy formulation
Notes
Sources
Further reading

In mathematics, the p-Laplacian, or the p-Laplace operator, is a quasilinear elliptic partial differential operator of 2nd order. It is a nonlinear generalization of the Laplace operator, where is allowed to range over 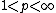. It is written as

Where the 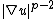is defined as

In the special case when , this operator reduces to the usual Laplacian.^[1] In general solutions of equations involving the p-Laplacian do not have second order derivatives in classical sense, thus solutions to these equations have to be understood as weak solutions. For example, we say that a function u belonging to the Sobolev space 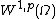is a weak solution of

if for every test function 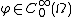we have

where denotes the standard scalar product.

Energy formulation

The weak solution of the p-Laplace equation with Dirichlet boundary conditions

in a domain 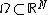is the minimizer of the energy functional

among all functions in the Sobolev space 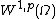satisfying the boundary conditions in the trace sense.^[1] In the particular case 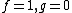and is a ball of radius 1, the weak solution of the problem above can be explicitly computed and is given by

where is a suitable constant depending on the dimension and on only. Observe that for the solution is not twice differentiable in classical sense.

Notes

1. ^¹Evans, pp 356.

Sources

{{cite journal | last = Evans | first = Lawrence C. | authorlink = Lawrence C. Evans | title = A New Proof of Local Regularity for Solutions of Certain Degenerate Elliptic P.D.E. | journal = Journal of Differential Equations | volume = 45 | pages = 356–373 | year = 1982| mr=672713 | doi=10.1016/0022-0396(82)90033-x}}
{{cite journal | last = Lewis | first= John L. | title = Capacitary functions in convex rings | journal = Archive for Rational Mechanics and Analysis | volume = 66 | pages = 201–224 | year = 1977|mr=0477094 | doi=10.1007/bf00250671}}

}}.

{{cite journal | last = Uhlenbeck | first= K. |author-link= Karen Uhlenbeck | title = Regularity for a class of non-linear elliptic systems | journal = Acta Mathematica | volume = 138 | pages = 219–240 | year = 1977|mr=0474389 | doi=10.1007/bf02392316}}
Notes on the p-Laplace equation by Peter Lindqvist
[https://www.scilag.net/problem/P-180730.1 Juan Manfredi, Strong comparison Principle for p-harmonic functions]

1 : Elliptic partial differential equations

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Energy formulation

Notes

Sources

Further reading