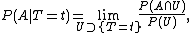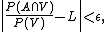“Regular conditional probability”的意思、由来-开放百科全书

Regular conditional probability is a concept that has developed to overcome certain difficulties in formally defining conditional probabilities for continuous probability distributions. It is defined as an alternative probability measure conditioned on a particular value of a random variable.

Motivation

Normally we define the conditional probability of an event A given an event B as:

The difficulty with this arises when the event B is too small to have a non-zero probability. For example, suppose we have a random variable X with a uniform distribution on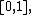

and B is the event that

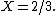Clearly, the probability of B, in this case, is

but nonetheless we would still like to assign meaning to a conditional probability such as

To do so rigorously requires the definition of a regular conditional probability.

Definition

Let

be a probability space, and let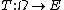

be a random variable, defined as a Borel-measurable function from

to its state space

Then a regular conditional probability is defined as a function

called a "transition probability", where

is a valid probability measure (in its second argument) on

for all

and a measurable function in E (in its first argument) for all

such that for all

and all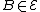

^[1]

where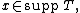

i.e. the topological support of the pushforward measure

As can be seen from the integral above, the value of

for points x outside the support of the random variable is meaningless; its significance as a conditional probability is strictly limited to the support of T.

The measurable space

is said to have the regular conditional probability property if for all probability measures

all random variables on

admit a regular conditional probability. A Radon space, in particular, has this property.

Alternate definition

Consider a Radon space

(that is a probability measure defined on a Radon space endowed with the Borel sigma-algebra) and a real-valued random variable T. As discussed above, in this case there exists a regular conditional probability with respect to T. Moreover, we can alternatively define the regular conditional probability for an event A given a particular value t of the random variable T in the following manner:

where the limit is taken over the net of open neighborhoods U of t as they become smaller with respect to set inclusion. This limit is defined if and only if the probability space is Radon, and only in the support of T, as described in the article. This is the restriction of the transition probability to the support of T. To describe this limiting process rigorously:

For every

there exists an open neighborhood U of the event {T=t}, such that for every open V with

Example

To continue with our motivating example above, we consider a real-valued random variable X and write

(where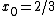

for the example given.) This limit, if it exists, is a regular conditional probability for X, restricted to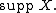

In any case, it is easy to see that this limit fails to exist for

outside the support of X: since the support of a random variable is defined as the set of all points in its state space whose every neighborhood has positive probability, for every point

outside the support of X (by definition) there will be an

such that

Thus if X is distributed uniformly on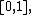

it is truly meaningless to condition a probability on "

Motivation

Definition

Alternate definition

Example

See also

References

External links