“Semi-infinite”的意思、由来-开放百科全书

In mathematics, semi-infinite objects are objects which are infinite or unbounded in some but not all possible ways.

In ordered structures and Euclidean spaces

Generally, a semi-infinite set is bounded in one direction, and unbounded in another. For instance, the natural numbers are semi-infinite considered as a subset of the integers; similarly, the intervals

and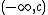

and their closed counterparts are semi-infinite subsets of

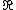. Half-spaces are sometimes described as semi-infinite regions.

Semi-infinite regions occur frequently in the study of differential equations.^[1]^[2] For instance, one might study solutions of the heat equation in an idealised semi-infinite metal bar.

A semi-infinite integral is an improper integral over a semi-infinite interval. More generally, objects indexed or parametrised by semi-infinite sets may be described as semi-infinite.^[3]

Most forms of semi-infiniteness are boundedness properties, not cardinality or measure properties: semi-infinite sets are typically infinite in cardinality and measure.

In optimization

Many optimization problems involve some set of variables and some set of constraints. A problem is called semi-infinite if one (but not both) of these sets is finite. The study of such problems is known as semi-infinite programming.^[4]

References

1. ^Bateman, Transverse seismic waves on the surface of a semi-infinite solid composed of heterogeneous material, Bull. Amer. Math. Soc. Volume 34, Number 3 (1928), 343–348.
2. ^Wolfram Demonstrations Project, Heat Diffusion in a Semi-Infinite Region (accessed November 2010).
3. ^Cator, Pimentel, [https://arxiv.org/abs/1001.4706v3 A shape theorem and semi-infinite geodesics for the Hammersley model with random weights], 2010.
4. ^Reemsten, Rückmann, [https://books.google.com/books?id=sJgX5jQZnQcC&lpg=PP1&ots=gvz6-MY_t1&dq=semi-infinite%20programming&pg=PP1#v=onepage&q&f=false Semi-infinite Programming], Kluwer Academic, 1998. {{ISBN|0-7923-5054-5}}