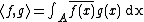“Square-integrable function”的意思、由来-开放百科全书

词条

Square-integrable function

释义

Properties
See also
References

{{Short description|function whose squared absolute value has finite integral}}

In mathematics, a square-integrable function, also called a quadratically integrable function, is a real- or complex-valued measurable function for which the integral of the square of the absolute value is finite. Thus, square-integrability on the real line 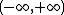is defined as follows.

{{Equation box 1
|indent =
|title=
|equation =

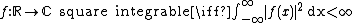
|cellpadding= 6
|border
|border colour = #0073CF
|background colour=#F5FFFA}}

One may also speak of quadratic integrability over bounded intervals such as for .^[1]

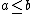{{Equation box 1
|indent =
|title=
|equation =

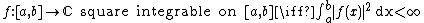
|cellpadding= 6
|border
|border colour = #0073CF
|background colour=#F5FFFA}}

An equivalent definition is to say that the square of the function itself (rather than of its absolute value) is Lebesgue integrable. For this to be true, the integrals of the positive and negative portions of the real part must both be finite, as well as those for the imaginary part. The vector space of square integrable functions (with respect to Lebesque measure) form the L^p -space with .

Often the term is used not to refer to a specific function, but to a set of functions that are equal almost everywhere.

Properties

The square integrable functions (in the sense mentioned in which a "function" actually means an equivalence class of functions that are equal almost everywhere) form an inner product space with inner product given by

where

and are square integrable functions,
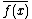is the complex conjugate of ,
is the set over which one integrates—in the first definition (given in the introduction above), is 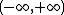; in the second, is .

Since 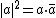, square integrability is the same as saying

It can be shown that square integrable functions form a complete metric space under the metric induced by the inner product defined above.

A complete metric space is also called a Cauchy space, because sequences in such metric spaces converge if and only if they are Cauchy.

A space which is complete under the metric induced by a norm is a Banach space.

Therefore, the space of square integrable functions is a Banach space, under the metric induced by the norm, which in turn is induced by the inner product.

As we have the additional property of the inner product, this is specifically a Hilbert space, because the space is complete under the metric induced by the inner product.

This inner product space is conventionally denoted by and many times abbreviated as .

Note that denotes the set of square integrable functions, but no selection of metric, norm or inner product are specified by this notation.

The set, together with the specific inner product 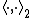specify the inner product space.

The space of square integrable functions is the L^p space in which .

References

1. ^{{cite book | title = Orthogonal Functions | author = G.Sansone | publisher = Dover Publications | year = 1991 | isbn = 978-0-486-66730-0 | pages = 1–2 }}

1 : Functional analysis

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Properties

See also

References