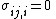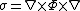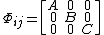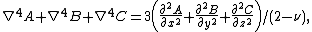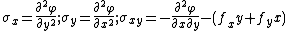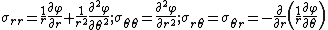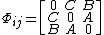“Stress functions”的意思、由来-开放百科全书

词条

Stress functions

释义

Beltrami stress functions
Maxwell stress functions
Airy stress function
Morera stress functions
Prandtl stress function
Notes
References
See also

In linear elasticity, the equations describing the deformation of an elastic body subject only to surface forces (&/or body forces that could be expressed as potentials) on the boundary are (using index notation) the equilibrium equation:

where is the stress tensor, and the Beltrami-Michell compatibility equations:

A general solution of these equations may be expressed in terms the Beltrami stress tensor. Stress functions are derived as special cases of this Beltrami stress tensor which, although less general, sometimes will yield a more tractable method of solution for the elastic equations.

Beltrami stress functions

It can be shown ^[1] that a complete solution to the equilibrium equations may be written as

Using index notation:

Engineering notation

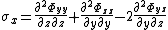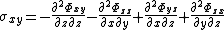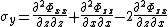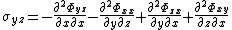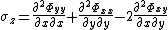where 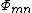is an arbitrary second-rank tensor field that is continuously differentiable at least four times, and is known as the Beltrami stress tensor.^[1] Its components are known as Beltrami stress functions. is the Levi-Civita pseudotensor, with all values equal to zero except those in which the indices are not repeated. For a set of non-repeating indices the component value will be +1 for even permutations of the indices, and -1 for odd permutations. And is the Nabla operator.

Maxwell stress functions

The Maxwell stress functions are defined by assuming that the Beltrami stress tensor 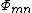is restricted to be of the form.^[2]

The stress tensor which automatically obeys the equilibrium equation may now be written as:^[2]

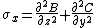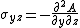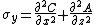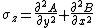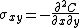The solution to the elastostatic problem now consists of finding the three stress functions which give a stress tensor which obeys the Beltrami–Michell compatibility equations for stress. Substituting the expressions for the stress into the Beltrami-Michell equations yields the expression of the elastostatic problem in terms of the stress functions:^[3]

These must also yield a stress tensor which obeys the specified boundary conditions.

Airy stress function

The Airy stress function is a special case of the Maxwell stress functions, in which it is assumed that A=B=0 and C is a function of x and y only.^[2] This stress function can therefore be used only for two-dimensional problems. In the elasticity literature, the stress function is usually represented by and the stresses are expressed as

Where 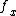and 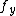are values of body forces in relevant direction.

In polar coordinates the expressions are:

Morera stress functions

The Morera stress functions are defined by assuming that the Beltrami stress tensor 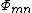tensor is restricted to be of the form ^[2]

The solution to the elastostatic problem now consists of finding the three stress functions which give a stress tensor which obeys the Beltrami-Michell compatibility equations. Substituting the expressions for the stress into the Beltrami-Michell equations yields the expression of the elastostatic problem in terms of the stress functions:^[4]

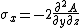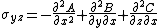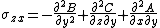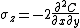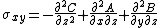Prandtl stress function

The Prandtl stress function is a special case of the Morera stress functions, in which it is assumed that A=B=0 and C is a function of x and y only.^[4]

Notes

1. ^¹{{cite book | last = Sadd | first = Martin H. | title = Elasticity: Theory, Applications, and Numerics | publisher = Elsevier Science & Technology Books | page = 363 | url = http://www.sciencedirect.com/science/book/9780126058116 | isbn =978-0-12-605811-6 }}
2. ^¹²³Sadd, M. H. (2005) Elasticity: Theory, Applications, and Numerics, Elsevier, p. 364
3. ^Knops (1958) p327
4. ^¹Sadd, M. H. (2005) Elasticity: Theory, Applications, and Numerics, Elsevier, p. 365

References

{{cite book |title=Elasticity - Theory, applications and numerics. |last=Sadd |first=Martin H. |year=2005 |publisher=Elsevier Butterworth-Heinemann |location=New York |isbn=0-12-605811-3 |oclc=162576656}}
{{cite journal |last=Knops |first=R. J. |year=1958|title=On the Variation of Poisson's Ratio in the Solution of Elastic Problems |journal=The Quarterly Journal of Mechanics and Applied Mathematics |volume=11 |issue=3 |pages=326–350 |doi=10.1093/qjmam/11.3.326 |url=http://qjmam.oxfordjournals.org/cgi/content/abstract/11/3/326|publisher=Oxford University Press }}