- Formula
- References
In numerical analysis, transfinite interpolation is a means to construct functions over a planar domain in such a way that they match a given function on the boundary. This method is applied in geometric modelling and in the field of finite element method.[1]
The transfinite interpolation method, first introduced by William J. Gordon and Charles A. Hall,[2] receives its name due to how a function belonging to this class is able to match the primitive function at a nondenumerable number of points.[3]
In the authors' words:
{{centered pull quote| We use the term ‘transfinite’ to describe the general class of interpolation schemes studied herein since, unlike the classical methods of higher dimensional interpolation which match the primitive function F at a finite number of distinct points, these methods match F at a non-denumerable (transfinite) number of points.}}Transfinite interpolation is similar to the Coons patch, invented in 1967. [1]
Formula
With parametrized curves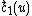 
, 
describing one pair of opposite sides of a domain, and
, 
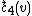describing the other pair. the position of point (u,v) in the domain is
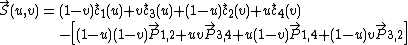
where, e.g., 
is the point where curves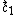 
and 
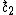meet.
References
- Croque, Newfoundland
- Croquette (film)
- Croquet Tintype
- Croron Mein Khel
- Crosato
- Crosbie, Andrew
- Crosbie, David
- Crosbie, James
- Crosbie, Richard
- Crosbie, William
- Crosby, Adam
- Crosby, Alabama
- Crosby, Andrew
- Crosbyarachne
- Crosby Beach, Minnesota
- Crosby, Brian
- Crosby by-election, 1953
- Crosby by-election, 1981
- Crosby, Columbo, and Vallee
- Crosby Crossing, Arizona
- Crosbycus dasycnemus
- Crosby, David
- Crosbyella
- Crosbyella distincta
- Crosbyella montana