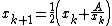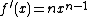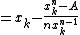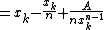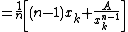“Nth root algorithm”的意思、由来-开放百科全书

词条

Nth root algorithm

释义

Derivation from Newton's method
See also
References

The principal nth root of a positive real number A, is the positive real solution of the equation

.

(For a positive integer n there are n distinct complex solutions to this equation if 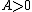, but only one is positive and real).

There is a very fast-converging nth root algorithm for finding :

Make an initial guess
Set 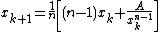. In practice we do .
Repeat step 2 until the desired precision is reached, i.e. .

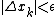A special case is the familiar square-root algorithm. By setting n = 2, the iteration rule in step 2 becomes the square root iteration rule:

Several different derivations of this algorithm are possible. One derivation shows it is a special case of Newton's method (also called the Newton-Raphson method) for finding zeros of a function beginning with an initial guess. Although Newton's method is iterative, meaning it approaches the solution through a series of increasingly accurate guesses, it converges very quickly. The rate of convergence is quadratic, meaning roughly that the number of bits of accuracy doubles on each iteration (so improving a guess from 1 bit to 64 bits of precision requires only 6 iterations). For this reason, this algorithm is often used in computers as a very fast method to calculate square roots.

For large n, the n^th root algorithm is somewhat less efficient since it requires the computation of at each step, but can be efficiently implemented with a good exponentiation algorithm.

Derivation from Newton's method

Newton's method is a method for finding a zero of a function f(x). The general iteration scheme is:

Make an initial guess
Set
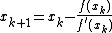
Repeat step 2 until the desired precision is reached.

The n^th root problem can be viewed as searching for a zero of the function

So the derivative is

and the iteration rule is

leading to the general n^th root algorithm.

References

{{Citation |first=Kendall E. |last=Atkinson |title=An introduction to numerical analysis |location=New York |publisher=Wiley |year=1989 |edition=2nd |isbn=0-471-62489-6 }}.

1 : Root-finding algorithms

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。

Derivation from Newton's method

See also

References