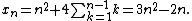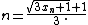1. Test for octagonal numbers

2. See also

3. References

An octagonal number is a figurate number that represents an octagon. The octagonal number for n is given by the formula 3n² - 2n, with n > 0. The first few octagonal numbers are:

1, 8, 21, 40, 65, 96, 133, 176, 225, 280, 341, 408, 481, 560, 645, 736, 833, 936 {{OEIS|id=A000567}}

Octagonal numbers can be formed by placing triangular numbers on the four sides of a square. To put it algebraically, the n-th octagonal number is

The octagonal number for n can also be calculated by adding the square of n to twice the (n - 1)th pronic number.

Octagonal numbers consistently alternate parity.

Octagonal numbers are occasionally referred to as "star numbers," though that term is more commonly used to refer to centered dodecagonal numbers.^[1]

Test for octagonal numbers

Solving the formula for the n-th octagonal number, for n gives

An arbitrary number x can be checked for octagonality by putting it in this equation. If n is an integer, then x is the n-th octagonal number. If n is not an integer, then x is not octagonal.

See also

• Centered octagonal number

References

• Eupithecia husseini
• Eupithecia hydrargyrea
• Eupithecia hypognampta
• Eupithecia hypophasma
• Eupithecia hysterica
• Eupithecia ichinosawana
• Eupithecia idaeoides
• Eupithecia idalia
• Eupithecia idonea
• Eupithecia illaborata
• Eupithecia illepidus
• Eupithecia illuminata
• Eupithecia immensa
• Eupithecia immixtaria
• Eupithecia immodica
• Eupithecia impavida
• Eupithecia implorata
• Eupithecia impolita
• Eupithecia importuna
• Eupithecia improba
• Eupithecia improvisa
• Eupithecia impurata
• Eupithecia ina
• Eupithecia inassignata
• Eupithecia incertata