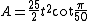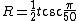“Pentacontagon”的意思、由来-开放百科全书

词条

Pentacontagon

释义

Regular pentacontagon properties
Symmetry
Dissection
Pentacontagram
References

In geometry, a pentacontagon or pentecontagon or 50-gon is a fifty-sided polygon.^[1]^[2] The sum of any pentacontagon's interior angles is 8640 degrees.

A regular pentacontagon is represented by Schläfli symbol {50} and can be constructed as a quasiregular truncated icosipentagon, t{25}, which alternates two types of edges.

Regular pentacontagon properties

One interior angle in a regular pentacontagon is 172{{frac|4|5}}°, meaning that one exterior angle would be 7{{frac|1|5}}°.

The area of a regular pentacontagon is (with {{nowrap|t {{=}} edge length}})

and its inradius is

The circumradius of a regular pentacontagon is

Since 50 = 2 × 5², a regular pentacontagon is not constructible using a compass and straightedge,^[3] and is not constructible even if the use of an angle trisector is allowed.^[4]

Symmetry

The regular pentacontagon has Dih₅₀ dihedral symmetry, order 100, represented by 50 lines of reflection. Dih₅₀ has 5 dihedral subgroups: Dih₂₅, (Dih₁₀, Dih₅), and (Dih₂, Dih₁). It also has 6 more cyclic symmetries as subgroups: (Z₅₀, Z₂₅), (Z₁₀, Z₅), and (Z₂, Z₁), with Z_n representing π/n radian rotational symmetry.

John Conway labels these lower symmetries with a letter and order of the symmetry follows the letter.^[5] He gives d (diagonal) with mirror lines through vertices, p with mirror lines through edges (perpendicular), i with mirror lines through both vertices and edges, and g for rotational symmetry. a1 labels no symmetry.

These lower symmetries allows degrees of freedom in defining irregular pentacontagons. Only the g50 subgroup has no degrees of freedom but can seen as directed edges.

Dissection

Coxeter states that every zonogon (a 2m-gon whose opposite sides are parallel and of equal length) can be dissected into m(m-1)/2 parallelograms.^[6]

In particular this is true for regular polygons with evenly many sides, in which case the parallelograms are all rhombi. For the regular pentacontagon, m=25, it can be divided into 300: 12 sets of 25 rhombs. This decomposition is based on a Petrie polygon projection of a 25-cube.

Pentacontagram

A pentacontagram is a 50-sided star polygon. There are 9 regular forms given by Schläfli symbols {50/3}, {50/7}, {50/9}, {50/11}, {50/13}, {50/17}, {50/19}, {50/21}, and {50/23}, as well as 16 compound star figures with the same vertex configuration.

Regular star polygons {50/k}
Picture	{{{frac>50\|3}}}	{{{frac>50\|7}}}	{{{frac>50\|9}}}	{{{frac>50\|11}}}	{{frac>50\|13}}
Interior angle	158.4°	129.6°	115.2°	100.8°	86.4°
Picture	{{{frac>50\|17}} }	{{{frac>50\|19}} }	{{{frac>50\|21}} }	{{{frac>50\|23}} }
Interior angle	57.6°	43.2°	28.8°	14.4°

References

1. ^{{citation|title=The Facts on File Geometry Handbook|first=Catherine A.|last=Gorini|publisher=Infobase Publishing|year=2009|isbn=9781438109572|page=120|url=https://books.google.com/books?id=PlYCcvgLJxYC&pg=PA120}}.
2. ^[https://books.google.com/books?ei=fpDlVLuCDNCYuQTEx4LwDA&id=wALvAAAAMAAJ&dq=pentecontagon&q=hebdomecontagon&redir_esc=y The New Elements of Mathematics: Algebra and Geometry] by Charles Sanders Peirce (1976), p.298
3. ^Constructible Polygon
4. ^{{cite web |url=http://www.math.iastate.edu/thesisarchive/MSM/EekhoffMSMSS07.pdf |title=Archived copy |accessdate=2015-02-19 |deadurl=yes |archiveurl=https://web.archive.org/web/20150714082609/http://www.math.iastate.edu/thesisarchive/MSM/EekhoffMSMSS07.pdf |archivedate=2015-07-14 |df= }}
5. ^The Symmetries of Things, Chapter 20
6. ^Coxeter, Mathematical recreations and Essays, Thirteenth edition, p.141

{{MathWorld|title=Pentacontagon|urlname=Pentacontagon}}
Naming Polygons and Polyhedra
[https://books.google.com/books?id=ugBDAAAAIAAJ&lpg=PA194&ots=eu5YMWxvXz&dq=pentecontagon&pg=PA194#v=onepage&q=pentecontagon&f=false Pentecontagon]

1 : Polygons

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。