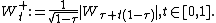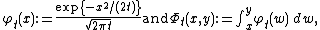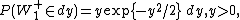- References
In the mathematical theory of probability, Brownian meander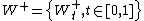 
is a continuous non-homogeneous Markov process defined as follows:
Let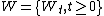 
be a standard one-dimensional Brownian motion, and 
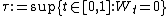, i.e. the last time before t = 1 when 
visits 
. Then the Brownian meander is defined by the following:
In words, let 
be the last time before 1 that a standard Brownian motion visits . (
almost surely.) We snip off and discard the trajectory of Brownian motion before , and scale the remaining part so that it spans a time interval of length 1. The scaling factor for the spatial axis must be square root of the scaling factor for the time axis. The process resulting from this snip-and-scale procedure is a Brownian meander. As the name suggests, it is a piece of Brownian motion that spends all its time away from its starting point .
The transition density 
of Brownian meander is described as follows:
For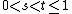 
and 
, and writing
we have
and
In particular,
i.e.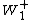 
has the Rayleigh distribution with parameter 1, the same distribution as 
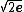, where 
is an exponential random variable with parameter 1.
References
- {{Cite journal |author1=Durett, Richard |author2=Iglehart, Donald |author3=Miller, Douglas |year= 1977|title= Weak convergence to Brownian meander and Brownian excursion | journal = The Annals of Probability | volume = 5 | issue = 1| pages = 117–129 |
url = http://projecteuclid.org/DPubS?service=UI&version=1.0&verb=Display&handle=euclid.aop/1176995895 | doi=10.1214/aop/1176995895}}
- {{Cite book |author1=Revuz, Daniel |author2=Yor, Marc |title=Continuous Martingales and Brownian Motion |edition=2nd |isbn=3-540-57622-3 |publisher=Springer-Verlag |location=New York |year=1999}}