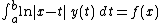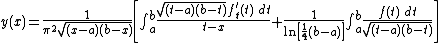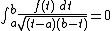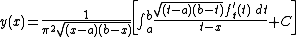- References
In mathematics, Carleman's equation is a Fredholm integral equation of the first kind with a logarithmic kernel. Its solution was first given by Torsten Carleman in 1922.
The equation is
The solution for b − a ≠ 4 is
If b − a = 4 then the equation is solvable only if the following condition is satisfied
In this case the solution has the form
where C is an arbitrary constant.
For the special case f(t) = 1 (in which case it is necessary to have b − a ≠ 4), useful in some applications, we get
References
- CARLEMAN, T. (1922) Uber die Abelsche Integralgleichung mit konstanten Integrationsgrenzen. Math. Z., 15, 111–120
- Gakhov, F. D., Boundary Value Problems [in Russian], Nauka, Moscow, 1977
- A.D. Polyanin and A.V. Manzhirov, Handbook of Integral Equations, CRC Press, Boca Raton, 1998. {{ISBN|0-8493-2876-4}}
2 : Fredholm theory|Integral equations
- World Open Gastroenterology Journal
- World Open Gastrointestinal Medicine Journal
- World Open Gastrointest. Med. J.
- World Open Gastrointest Med J
- World Open Gels J
- World Open Gels J.
- World Open Gels Journal
- World Open Gender and Sexuality Studies Journal
- World Open Gender and Sustainable Development Journal
- World Open Gender & Sexuality Studies Journal
- World Open Gender & Sustainable Development Journal
- World Open Gend Sex Stud J
- World Open Gend. Sex. Stud. J.
- World Open Gend. Sustain. Dev. J.
- World Open Gend Sustain Dev J
- World Open General Medicine Journal
- World Open General Practice Journal
- World Open General Science Journal
- World Open Genet Eng Biotechnol J
- World Open Genet. Eng. Biotechnol. J.
- World Open Genet. Eng. J.
- World Open Genet Eng J
- World Open Gene Therapy Journal
- World Open Gene Ther J
- World Open Gene Ther. J.