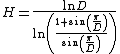“Draft:Fractal space map”的意思、由来-开放百科全书

A fractal space map, or as they later became known, simply a fractal map, uses a fractal concept to allow large quantities of hierarchical data to be displayed on a computer screen in a clear manner.

The original patent applications^[1]^[2] filed on this technique refer to a number of alternative iterated function systems that can be used for this purpose. One such, for example, is similar to a Sierpinski triangle and another, intended primarily for use in conjunction with a telephone keypad, resembles a Sierpinski carpet or Cantor dust. The preferred form of fractal identified, however, and almost uniformly adopted thereafter for practical purposes, was based on a circular pattern that differed from previously known circle-based fractals such as the Apollonian gasket in that it did not seek to pack the available space so tightly.

Advantages

Whereas a fractal based on triangles, squares or any shape with corners only provides an unbiased representation of a hierarchy when the degree of the hierarchy matches a multiple of the number of the shape's vertices, a Fractal Map based on circles successfully adapts to represent hierarchies with any number of branches – see the Fractal Map with various degrees diagram opposite.

Whereas the tighter packing exhibited by an Apollonian gasket seems at first glance that it might provide a more efficient layout than a Fractal Map based on circles, when the ease of user interpretation and manipulation of the system are taken into account, leaving more screen real estate dedicated to the representation of higher level nodes in the hierarchy has significant advantages. It creates a clearer sense of the structure of the hierarchy as well as providing areas which can be easily selected to drill down into the hierarchy. Perhaps most subtly, if child regions are arranged around the periphery of their parent region, it also allows a labelling system to be implemented that can successfully display the labels for several nodes at once – see the Fractal Map labelling diagram opposite. This is not possible with other systems that seek to fill the available space too tightly.

These advantages also hold true when comparing Fractal Maps to TreeMaps, another way, though not strictly fractal, of representing hierarchical data.

Applications

Hierarchies are extremely common throughout business, science, government, education and indeed every day life. Product categories, industry sectors and sub sectors, organizational hierarchies, species classifications, family trees ... the list goes on and on. It should not come as any surprise, therefore, that Fractal Maps can be applied to improve data access in a wide range of situations. The efficient layout of Fractal Maps is often combined with the well-known concept of a heat map to allow users to identify outliers and trends in the underlying variables associated with nodes in the hierarchy. See, for example, the Fractal Map of global equity markets diagram opposite.

Construction and dimension

For an evenly weighted Fractal Map based on circles, given a parent circle of radius R and N child circles, the radius of the child circles, r, is given by the following formula:

Therefore the Hausdorff dimension H of such a fractal map with fixed degree D, is given by the following formula:

Thus the Hausdorff dimension of a fixed-degree fractal map is greatest when the degree is 6. When the degree is 3, the Hausdorff dimension is approximately 1.6309, slightly greater than 1.5485, the approximate Hausdorff dimension of a Sierpinski triangle.

References

1. ^{{cite book |last=Clifton-Bligh |first=Gervase |title=Mapping Logically Related Data Files |year=1998 |publisher=European Patent Office |url=http://wo.espacenet.com/publicationDetails/biblio?CC=WO&NR=0013103A1&KC=A1&FT=D&date=20000309&DB=wo.espacenet.com&locale=en_EP |ref=WO13103}}
2. ^{{cite book |last=Clifton-Bligh |first=Gervase |title=Methods and Devices for Mapping Data Files |year=1999 |publisher=European Patent Office |url=http://wo.espacenet.com/publicationDetails/biblio?CC=WO&NR=0013104A1&KC=A1&FT=D&date=20000309&DB=wo.espacenet.com&locale=en_EP |ref=WO13104}}

Advantages

Applications

Construction and dimension

References

External links