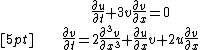“Drinfeld–Sokolov–Wilson equation”的意思、由来-开放百科全书

The Drinfeld–Sokolov–Wilson (DSW) equations are an integrable system of two coupled nonlinear partial differential equations proposed by Vladimir Drinfeld and Vladimir Sokolov, and independently by George Wilson:^[1]^[2]

Notes

1. ^{{cite journal |author=Esmaeil Alibeiki and Ahmad Neyrameh |title=Application of Homotopy Perturbation Method to Nonlinear Drinfeld–Sokolov–Wilson Equation |year=2011 |journal=Middle-East Journal of Scientific Research |volume=10 |issue=4 |pages=440–443 |url=http://idosi.org/mejsr/mejsr10(4)11/3.pdf}}
2. ^{{MathWorld |id=Drinfeld-Sokolov-WilsonEquation |title=Drinfeld–Sokolov–Wilson Equation |author=Weisstein, Eric W.}}

References

Graham W. Griffiths, William E. Shiesser Traveling Wave Analysis of Partial Differential Equations, p. 135 Academy Press

Richard H. Enns, George C. McCGuire, Nonlinear Physics Birkhauser, 1997

Inna Shingareva, Carlos Lizárraga-Celaya, Solving Nonlinear Partial Differential Equations with Maple Springer.

Eryk Infeld and George Rowlands, Nonlinear Waves,Solitons and Chaos, Cambridge 2000

Saber Elaydi, An Introduction to Difference Equations, Springer 2000

Dongming Wang, Elimination Practice, Imperial College Press 2004

David Betounes, Partial Differential Equations for Computational Science: With Maple and Vector Analysis Springer, 1998 {{ISBN|9780387983004}}

George Articolo, Partial Differential Equations & Boundary Value Problems with Maple V, Academic Press 1998 {{ISBN|9780120644759}}

2 : Nonlinear partial differential equations|Integrable systems