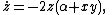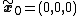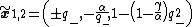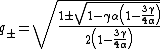“Rabinovich–Fabrikant equations”的意思、由来-开放百科全书

词条

Rabinovich–Fabrikant equations

释义

System description
Equilibrium points γ = 0.87, α = 1.1 γ = 0.1
See also
References
External links

The Rabinovich–Fabrikant equations are a set of three coupled ordinary differential equations exhibiting chaotic behavior for certain values of the parameters. They are named after Mikhail Rabinovich and Anatoly Fabrikant, who described them in 1979.

System description

The equations are:^[1]

where α, γ are constants that control the evolution of the system. For some values of α and γ, the system is chaotic, but for others it tends to a stable periodic orbit.

Danca and Chen^[2] note that the Rabinovich–Fabrikant system is difficult to analyse (due to the presence of quadratic and cubic terms) and that different attractors can be obtained for the same parameters by using different step sizes in the integration. Also, recently, a hidden attractor was discovered in the Rabinovich–Fabrikant system ^[1].

Equilibrium points

The Rabinovich–Fabrikant system has five hyperbolic equilibrium points, one at the origin and four dependent on the system parameters α and γ:^[2]

where

These equilibrium points only exist for certain values of α and γ > 0.

γ = 0.87, α = 1.1

An example of chaotic behavior is obtained for γ = 0.87 and α = 1.1 with initial conditions of (−1, 0, 0.5).^[5] The correlation dimension was found to be 2.19 ± 0.01.^[6] The Lyapunov exponents, λ are approximately 0.1981, 0, −0.6581 and the Kaplan–Yorke dimension, D_KY ≈ 2.3010^[5]

γ = 0.1

Danca and Romera^[8] showed that for γ = 0.1, the system is chaotic for α = 0.98, but progresses on a stable limit cycle for α = 0.14.

References

^[2]^[3]^[4]^[5]^[6]

External links

Weisstein, Eric W. "Rabinovich–Fabrikant Equation." From MathWorld—A Wolfram Web Resource.
Chaotics Models a more appropriate approach to the chaotic graph of the system "Rabinovich–Fabrikant Equation"

2 : Chaotic maps|Equations

随便看