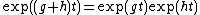- Construction
- Examples
- References
In mathematics, an exp algebra is a Hopf algebra Exp(G) constructed from an abelian group G, and is the universal ring R such that there is an exponential map from G to the group of the power series in R[[t]] with constant term 1. In other words the functor Exp from abelian groups to commutative rings is adjoint to the functor from commutative rings to abelian groups taking a ring to the group of formal power series with constant term 1.
The definition of the exp ring of G is similar to that of the group ring Z[G] of G, which is the universal ring such that there is an exponential homomorphism from the group to its units. In particular there is a natural homomorphism from the group ring to a completion of the exp ring. However in general the Exp ring can be much larger than the group ring: for example, the group ring of the integers is the ring of Laurent polynomials in 1 variable, while the exp ring is a polynomial ring in countably many generators.
Construction
For each element g of G introduce a countable set of variables gi for i>0. Define exp(gt) to be the formal power series in t
The exp ring of G is the commutative ring generated by all the elements gi with the relations
for all g, h in G; in other words the coefficients of any power of t on both sides are identified.
The ring Exp(G) can be made into a commutative and cocommutative Hopf algebra as follows. The coproduct of Exp(G) is defined so that all the elements exp(gt) are group-like. The antipode is defined by making exp(–gt) the antipode of exp(gt). The counit takes all the generators gi to 0.
{{harvtxt|Hoffman|1983}} showed that Exp(G) has the structure of a λ-ring.Examples
- The exp ring of an infinite cyclic group such as the integers is a polynomial ring in a countable number of generators gi where g is a generator of the cyclic group. This ring (or Hopf algebra) is naturally isomorphic to the ring of symmetric functions (or the Hopf algebra of symmetric functions).
- {{harvs|txt | MR=2724822 | zbl=1211.16023
|last=Hazewinkel|first= Michiel|last2= Gubareni|first2= Nadiya|last3= Kirichenko|first3= V. V.
|title=Algebras, rings and modules.
Lie algebras and Hopf algebras|series= Mathematical Surveys and Monographs|volume= 168|publisher= American Mathematical Society|place= Providence, RI|year= 2010|ISBN= 978-0-8218-5262-0 }} suggest that it might be interesting to extend the theory to non-commutative groups G.
References
- {{citation | MR=2724822 | zbl=1211.16023
|last=Hazewinkel|first= Michiel|last2= Gubareni|first2= Nadiya|last3= Kirichenko|first3= V. V.
|title=Algebras, rings and modules. Lie algebras and Hopf algebras|series= Mathematical Surveys and Monographs|volume= 168|publisher= American Mathematical Society|place= Providence, RI|year= 2010|ISBN= 978-0-8218-5262-0 }}
- {{citation|mr=0687747
|last=Hoffman|first= P.
|title=Exponential maps and λ-rings
|journal=J. Pure Appl. Algebra|volume= 27 |year=1983|issue= 2|pages= 131–162|doi=10.1016/0022-4049(83)90011-7}}