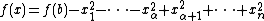“Gradient-like vector field”的意思、由来-开放百科全书

词条

Gradient-like vector field

释义

Definition
Dynamical system
References

In differential topology, a mathematical discipline, and more specifically in Morse theory, a gradient-like vector field is a generalization of gradient vector field.

The primary motivation is as a technical tool in the construction of Morse functions, to show that one can construct a function whose critical points are at distinct levels. One first constructs a Morse function, then uses gradient-like vector fields to move around the critical points, yielding a different Morse function.

Definition

Given a Morse function f on a manifold M, a gradient-like vector field X for the function f is, informally:

away from critical points, X points "in the same direction as" the gradient of f, and
near a critical point (in the neighborhood of a critical point), it equals the gradient of f, when f is written in standard form given in the Morse lemmas.

Formally:^[1]

away from critical points,
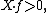
around every critical point there is a neighborhood on which f is given as in the Morse lemmas:

and on which X equals the gradient of f.

Dynamical system

The associated dynamical system of a gradient-like vector field, a gradient-like dynamical system, is a special case of a Morse–Smale system.

References

1. ^[https://books.google.com/books?id=TtKyqozvgIwC&pg=PA63 p. 63]

An introduction to Morse theory, Yukio Matsumoto, 2002, Section 2.3: Gradient-like vector fields, [https://books.google.com/books?id=TtKyqozvgIwC&pg=PA56 p. 56–69]
Gradient-Like Vector Fields Exist, September 25, 2009

2 : Morse theory|Differential topology

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。