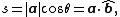1. Definition based on angle θ

2. Definition in terms of a and b

3. Properties

4. See also

In mathematics, the scalar projection of a vector on (or onto) a vector , also known as the scalar resolute of in the direction of , is given by:

where the operator denotes a dot product, is the unit vector in the direction of , is the length of , and is the angle between and .

The term scalar component refers sometimes to scalar projection, as, in Cartesian coordinates, the components of a vector are the scalar projections in the directions of the coordinate axes.

The scalar projection is a scalar, equal to the length of the orthogonal projection of on , with a negative sign if the projection has an opposite direction with respect to .

Multiplying the scalar projection of on by converts it into the above-mentioned orthogonal projection, also called vector projection of on .

Definition based on angle θ

If the angle between and is known, the scalar projection of on can be computed using

( in the figure)

Definition in terms of a and b

When is not known, the cosine of can be computed in terms of and , by the following property of the dot product :

By this property, the definition of the scalar projection 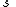becomes:

Properties

The scalar projection has a negative sign if 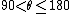degrees. It coincides with the length of the corresponding vector projection if the angle is smaller than 90°. More exactly, if the vector projection is denoted and its length :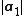

if degrees,

if degrees.

See also

• Scalar product
• Cross product
• Vector projection

• Clustered planarity
• Clustered regularly interspaced short palindromic repeat
• Clustered Regularly Interspaced Short Palindromic Repeat
• Clustered Regularly Interspaced Short Palindromic Repeats
• Clustered regularly interspaced short palindromic repeats
• Clustered settlement
• Clustered tarweed
• Clustered thistle
• Clustered village
• Clustered yellowtops
• Cluster (epidemiology)
• Cluster expansion approach
• Cluster-expansion approach
• Cluster flies
• Cluster-flower Geebung
• Cluster formation
• Cluster Frenzy
• Cluster hypothesis
• Cluster II
• Cluster II (disambiguation)
• Clustering of composers
• Cluster Innovation Centre
• Clusterization
• Cluster Lensing And Supernova survey with Hubble
• Cluster Lensing and Supernova survey with Hubble