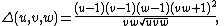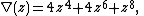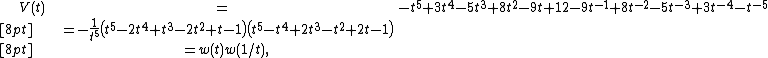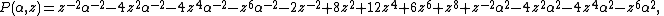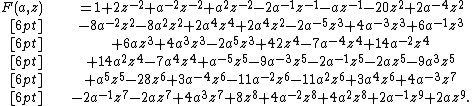“L10a140 link”的意思、由来-开放百科全书

词条

L10a140 link

释义

Invariants
Pseudo-symmetric visual variants
References
External links

{{Infobox knot theory
| name= L10a140
| practical name=
| image= Brunnian-L10a140.svg
| caption=
| arf invariant=
| braid length= 10
| braid number= 3
| bridge number=
| crossing number= 10
| hyperbolic volume= 12.27627758
| linking number=
| stick number=
| unknotting number=
| conway_notation=[.3:30]
| ab_notation=
| dowker notation=
| thistlethwaite= L10a140
| other=
| alternating= alternating
| fibered=
| slice=
| symmetry=
| tricolorable=
| last knot= L10a139
| next knot= L10a141
}}

In the mathematical theory of knots, L10a140 is the name in the Thistlewaite link table of a link of three loops, which has ten crossings between the loops when presented in its simplest visual form.^[1] It is of interest because it is presumably the simplest link which possesses the Brunnian property — a link of connected components that, when one component is removed, becomes entirely unconnected^[2] — other than the six-crossing Borromean rings.^[3]

In other words, no two loops are directly linked with each other, but all three are collectively interlinked, so removing any loop frees the other two. In the image in the infobox at right, the red loop is not interlinked with either the blue or the yellow loops, and if the red loop is removed, then the blue and yellow loops can also be disentangled from each other without cutting either one.

According to work by Slavik V. Jablan, the L10a140 link can be seen as the second in an infinite series of Brunnian links beginning with the Borromean rings. So if the blue and yellow loops have only one twist along each side, the resulting configuration is the Borromean rings; if the blue and yellow loops have three twists along each side, the resulting configuration is the L10a140 link; if the blue and yellow loops have five twists along each side, the resulting configuration is a three-loop link with 14 overall crossings, etc. etc.^[4]

Invariants

The multivariable Alexander polynomial for the L10a140 link is

the Conway polynomial is

the Jones polynomial factors nicely as

where 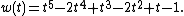(Notice that is essentially the Jones polynomial for the Whitehead link.)

The HOMFLY polynomial is

and the Kauffman polynomial is

Pseudo-symmetric visual variants

David Swart,^[5] and independently Rick Mabry and Laura McCormick,^[6] discovered alternative 12-crossing visual representations of the L10a140 link. In these depictions, the link no longer has strictly alternating crossings (as it does in its simplest 10-crossing form), but there is greater superficial symmetry.

So the leftmost image below shows a 12-crossing link (distinct from both the Borromean rings and the L10a140 link) with six-fold rotational symmetry. The center image shows a similar-looking depiction of the L10a140 link (but without true rotational symmetry). Similarly, the rightmost image shows a depiction of the L10a140 link with superficial fourfold symmetry.

References

1. ^{{Knot Atlas|L10a140}}
2. ^Adams, Colin C. (1994). The Knot Book, {{page needed|date=April 2013}}. American Mathematical Society. {{isbn|9780716723936}}.
3. ^Bar-Natan, Dror (2010-08-16). "All Brunnians, Maybe", Academic Pensieve.
4. ^Jablan, Slavik V., Are Borromean Links So Rare?, Forma 14 (1999), 269–277. Online at the electronic journal Vismath. L10a140 is depicted in the middle figure of the top image.
5. ^Dror Bar-Natan (2010-08-14). "A Link from David Swart", [Academic Pensieve].
6. ^{{cite journal |last=Swart|first=David |title=It is what it is |journal=Math Horizons |volume=18|issue=4 |date=April 2011}}

External links

[https://www.flickr.com/photos/dmswart/4878510547/ "It Is What It Is"], Flickr.com.

1 : Hyperbolic knots and links

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。