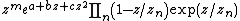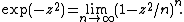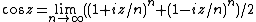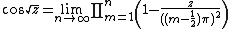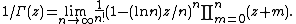“Laguerre–Pólya class”的意思、由来-开放百科全书

词条

Laguerre–Pólya class

释义

Examples
References

The Laguerre–Pólya class is the class of entire functions consisting of those functions which are locally the limit of a series of polynomials whose roots are all real.

^[1]

Any function of Laguerre–Pólya class is also of Pólya class.

The product of two functions in the class is also in the class, so the class constitutes a monoid under the operation of function multiplication.

Some properties of a function in the Laguerre–Pólya class are:

All roots are real.
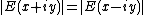for x and y real.
is a non-decreasing function of y for positive y.

A function is of Laguerre–Pólya class if and only if three conditions are met:

The roots are all real.
The nonzero zeros z_n satisfy

converges, with zeros counted according to their multiplicity)

The function can be expressed in the form of a Hadamard product

with b and c real and c non-positive. (The non-negative integer m will be positive if E(0)=0. Note that if the number of zeros is infinite one may have to define how to take the infinite product.)

Examples

Some examples are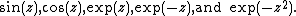

On the other hand, 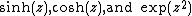are not in the Laguerre–Pólya class.

For example,

Cosine can be done in more than one way. Here is one series of polynomials having all real roots:

And here is another:

This shows the buildup of the Hadamard product for cosine.

If we replace z² with z, we have another function in the class:

Another example is the reciprocal gamma function 1/Γ(z). It is the limit of polynomials as follows:

References

1. ^"Approximation by entire functions belonging to the Laguerre–Pólya class" {{webarchive|url=https://web.archive.org/web/20081006131503/http://www.intlpress.com/MAA/p/1999/6_1/MAA-6-1-021-038.pdf |date=2008-10-06 }} by D. Dryanov and Q. I. Rahman, Methods and Applications of Analysis" 6 (1) 1999, pp. 21–38.

1 : Analytic functions

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。