“Sedenion”的意思、由来-开放百科全书

词条

Sedenion

释义

Arithmetic
Sedenion properties Anti-associative Quaternionic subalgebras
Applications
See also
Notes
References

{{Infobox number system
| official_name = Sedenions
| symbol =

| type = nonassociative algebra
| units = e₀...e₁₅
| identity = e₀
| properties = power associativity
distributivity
}}

In abstract algebra, the sedenions form a 16-dimensional noncommutative and nonassociative algebra over the reals; they are obtained by applying the Cayley–Dickson construction to the octonions, and as such the octonions are a subfield of the sedenions. Unlike the octonions, the sedenions are not an alternative algebra. Applying the Cayley-Dickson construction to the sedenions yields a 32-dimensional algebra, sometimes called the 32-ions or Trigintaduonions.^[1] It is possible to apply the Cayley-Dickson construction to the sedenions infinitely often.

The term sedenion is also used for other 16-dimensional algebraic structures, such as a tensor product of two copies of the biquaternions, or the algebra of 4 by 4 matrices over the reals, or that studied by {{harvtxt|Smith |1995}}.

Arithmetic

Like octonions, multiplication of sedenions is neither commutative nor associative.

But in contrast to the octonions, the sedenions do not even have the property of being alternative.

They do, however, have the property of power associativity, which can be stated as that, for any element x of , the power is well defined. They are also flexible.

Every sedenion is a linear combination of the unit sedenions , , , , ...,,

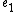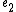which form a basis of the vector space of sedenions. Every sedenion can be represented in the form

.

Addition and subtraction are defined by the addition and subtraction of corresponding coefficients and multiplication is distributive over addition.

Like other algebras based on the Cayley–Dickson construction, the sedenions contain the algebra they were constructed from. So, they contain the octonions ( to 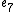in the table below), and therefore also the quaternions ( to ), complex numbers ( and 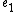) and reals ().

The sedenions have a multiplicative identity element and multiplicative inverses but they are not a division algebra because they have zero divisors. This means that two non-zero sedenions can be multiplied to obtain zero: an example is ( + )( 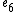− ). All hypercomplex number systems after sedenions that are based on the Cayley–Dickson construction contain zero divisors.

A sedenion multiplication table is shown below:

multiplier
! ! ! ! ! ! ! ! ! ! ! ! ! ! ! ! !
multiplicand	>	>	>	>	>	>	>	>	>	>	>	>	>	>	>	>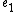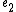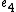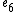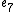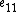
		−		−		−	−			−	−		−			−
		−	−				−	−			−	−	−	−
			−	−		−		−		−		−	−		−
		−	−	−	−								−	−	−	−
			−		−	−	−			−		−		−		−
				−	−		−	−		−	−			−	−
		−			−	−		−			−	−			−	−
		−	−	−	−	−	−	−	−
			−		−			−	−	−	−		−			−
				−	−	−			−		−	−	−	−
		−			−		−		−	−		−	−		−
						−	−	−	−				−	−	−	−
		−		−				−	−	−		−		−		−
		−	−			−			−	−	−			−	−
			−	−			−		−		−	−			−	−

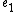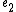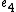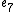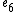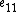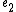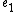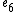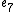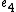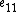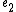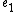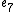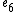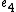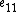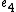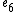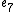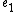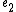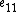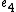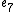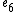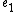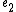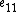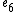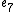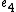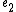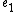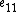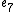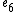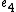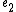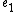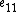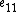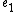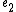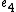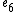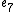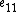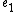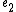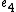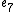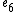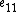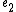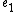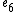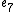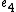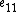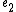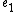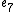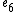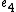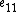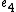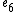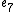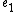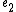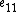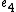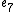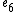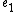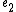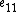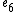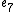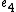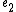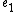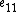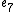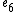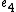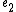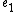Sedenion properties

From the above table, we can see that:

Anti-associative

The sedenions are NOT fully anti-associative. Choose any four generators, and . The following 5-cycle shows that at least one of these relations must associate.

In particular, in the table above, using 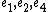and the last expression associates.

Quaternionic subalgebras

The 35 triads that make up this specific sedenion multiplication table with the 7 triads of the octonions used in creating the sedenion through the Cayley–Dickson construction shown in bold red:

The binary representations of the indices of these triples xor to 0.

{{{red|{1, 2, 3} }}, {{red|{1, 4, 5} }}, {{red|{1, 7, 6} }}, {1, 8, 9}, {1, 11, 10}, {1, 13, 12}, {1, 14, 15},
{{red|{2, 4, 6} }}, {{red|{2, 5, 7} }}, {2, 8, 10}, {2, 9, 11}, {2, 14, 12}, {2, 15, 13}, {{red|{3, 4, 7} }},
{{red|{3, 6, 5} }}, {3, 8, 11}, {3, 10, 9}, {3, 13, 14}, {3, 15, 12}, {4, 8, 12}, {4, 9, 13},
{4, 10, 14}, {4, 11, 15}, {5, 8, 13}, {5, 10, 15}, {5, 12, 9}, {5, 14, 11}, {6, 8, 14},
{6, 11, 13}, {6, 12, 10}, {6, 15, 9}, {7, 8, 15}, {7, 9, 14}, {7, 12, 11}, {7, 13, 10}}

The list of 84 sets of zero divisors {, , , }, where

( + )( + )=0:

Applications

{{harvtxt|Moreno|1998}} showed that the space of pairs of norm-one sedenions that multiply to zero is homeomorphic to the compact form of the exceptional Lie group G₂. (Note that in his paper, a "zero divisor" means a pair of elements that multiply to zero.)

Notes

1. ^Raoul E. Cawagas, et al. (2009). [https://arxiv.org/pdf/0907.2047.pdf "THE BASIC SUBALGEBRA STRUCTURE OF THE CAYLEY-DICKSON ALGEBRA OF DIMENSION 32 (TRIGINTADUONIONS)"].
2. ^{{Harv|Baez|2002|loc=p. 6}}

References

{{Citation | last1=Imaeda | first1=K. | last2=Imaeda | first2=M. | title=Sedenions: algebra and analysis | doi=10.1016/S0096-3003(99)00140-X | mr=1786945 | year=2000 | journal=Applied Mathematics and Computation | volume=115 | issue=2 | pages=77–88}}
{{Cite journal | ref = harv| last1 = Baez | first1 = John C. | authorlink = John Baez| title = The Octonions | journal = Bulletin of the American Mathematical Society |series=New Series | volume = 39 | issue = 2 | pages = 145–205 | year = 2002 | url = http://math.ucr.edu/home/baez/octonions/ | doi = 10.1090/S0273-0979-01-00934-X | arxiv = math/0105155v4| mr = 1886087| bibcode = 1994BAMaS..30..205W }}
{{cite journal |first=M.K. |last=Kinyon |last2=Phillips |first2=J.D. |last3=Vojtěchovský |first3=P. |title=C-loops: Extensions and constructions |journal=Journal of Algebra and its Applications |volume=6 |issue=1 |pages=1–20 |year=2007 |doi=10.1142/S0219498807001990 |arxiv=math/0412390|citeseerx=10.1.1.240.6208 }}
{{cite journal |first=Benard M. |last=Kivunge |last2=Smith |first2=Jonathan D. H |title=Subloops of sedenions |journal=Comment. Math. Univ. Carolinae |volume=45 |issue=2 |pages=295–302 |year=2004 |doi= |url=http://www.emis.de/journals/CMUC/pdf/cmuc0402/kivunge.pdf |format=PDF}}
{{Citation | last1=Moreno | first1=Guillermo | title=The zero divisors of the Cayley–Dickson algebras over the real numbers | arxiv=q-alg/9710013 | mr=1625585 | year=1998 | journal= Bol. Soc. Mat. Mexicana |series=Series 3| volume=4 | issue=1 | pages=13–28| bibcode=1997q.alg....10013G }}
{{Citation | last1=Smith | first1=Jonathan D. H. | title=A left loop on the 15-sphere | doi=10.1006/jabr.1995.1237 | mr=1345298 | year=1995 | journal=Journal of Algebra | volume=176 | issue=1 | pages=128–138}}

2 : Hypercomplex numbers|Non-associative algebras

随便看

开放百科全书收录14589846条英语、德语、日语等多语种百科知识，基本涵盖了大多数领域的百科知识，是一部内容自由、开放的电子版国际百科全书。