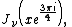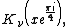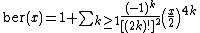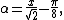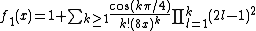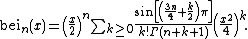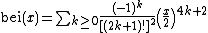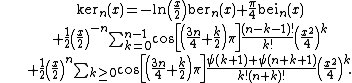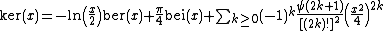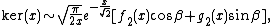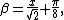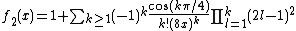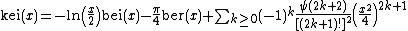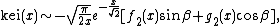“Kelvin functions”的意思、由来-开放百科全书

词条

Kelvin functions

释义

ber(x)
bei(x)
ker(x)
kei(x)
See also
References
External links

In applied mathematics, the Kelvin functions ber_ν(x) and bei_ν(x) are the real and imaginary parts, respectively, of

where x is real, and {{math|J_ν(z)}}, is the ν^th order Bessel function of the first kind. Similarly, the functions Ker_ν(x) and Kei_ν(x) are the real and imaginary parts, respectively, of

where {{math|K_ν(z)}} is the ν^th order modified Bessel function of the second kind.

These functions are named after William Thomson, 1st Baron Kelvin.

While the Kelvin functions are defined as the real and imaginary parts of Bessel functions with x taken to be real, the functions can be analytically continued for complex arguments {{math|xe^iφ, 0 ≤ φ < 2π.}} With the exception of Ber_n(x) and Bei_n(x) for integral n, the Kelvin functions have a branch point at x = 0.

Below, {{math|Γ(z)}} is the gamma function and {{math|ψ(z)}} is the digamma function.

ber(x)

For integers n, ber_n(x) has the series expansion

where {{math|Γ(z)}} is the gamma function. The special case ber₀(x), commonly denoted as just ber(x), has the series expansion

and asymptotic series

,

where

bei(x)

For integers n, bei_n(x) has the series expansion

The special case bei₀(x), commonly denoted as just bei(x), has the series expansion

and asymptotic series

where α, 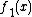, and 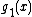are defined as for ber(x).

ker(x)

For integers n, ker_n(x) has the (complicated) series expansion

The special case ker₀(x), commonly denoted as just ker(x), has the series expansion

and the asymptotic series

where

kei(x)

For integer n, kei_n(x) has the series expansion

The special case kei₀(x), commonly denoted as just kei(x), has the series expansion

and the asymptotic series

where β, f₂(x), and g₂(x) are defined as for ker(x).

References

{{Abramowitz_Stegun_ref|9|379}}
{{dlmf|first=F. W. J. |last=Olver|first2=L. C. |last2=Maximon|id=10|title=Bessel functions}}

External links

Weisstein, Eric W. "Kelvin Functions." From MathWorld—A Wolfram Web Resource.
GPL-licensed C/C++ source code for calculating Kelvin functions at codecogs.com:

2 : Special hypergeometric functions|William Thomson, 1st Baron Kelvin

随便看