- Applications
- See also
- References Sources
In mathematics, the Langlands decomposition writes a parabolic subgroup P of a semisimple Lie group as a product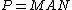 
of a reductive subgroup M, an abelian subgroup A, and a nilpotent subgroup N.
Applications
{{see also|Parabolic induction}}A key application is in parabolic induction, which leads to the Langlands program: if 
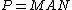is a reductive algebraic group and is the Langlands decomposition of a parabolic subgroup P, then parabolic induction consists of taking a representation of 
, extending it to 
by letting 
act trivially, and inducing the result from to .
See also
- Lie group decompositions
References
Sources
- A. W. Knapp, Structure theory of semisimple Lie groups. {{isbn|0-8218-0609-2}}.
2 : Lie groups|Algebraic groups
- Teneale Hatton
- Tene-angopte
- Tenebrae factae sunt
- Tenebrae Factae Sunt
- Tenebrae music
- Tenebrae responsoria
- Tenebrae Responsoria
- Tenebrae Responsoria (Gesualdo)
- Tenebrae Responsories
- Tenebrae responsories
- Tenebrae Responsories (Gesualdo)
- Tenebrae responsories, Op. 15 (Gesualdo)
- Tenebrae Responsories (Victoria)
- Tenebrae Responsory
- Tenebrae responsory
- Tenebrae Vision
- Tenebre (film)
- Tenebrimycin
- Tenebriso
- Tenebros
- Tenebrosa
- Tenebrous Liar
- Tenebrous worm
- Tenedla
- Ten'edn