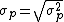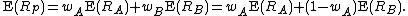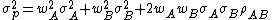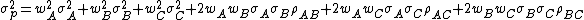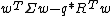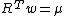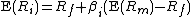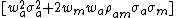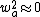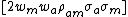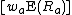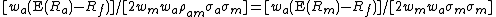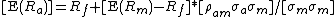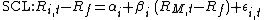- Mathematical model Risk and expected return Diversification Efficient frontier with no risk-free asset Two mutual fund theorem Risk-free asset and the capital allocation line
- Asset pricing Systematic risk and specific risk Capital asset pricing model
- Criticisms
- Extensions
- Connection with rational choice theory
- Other applications Project portfolios and other "non-financial" assets
- See also
- References
- Further reading
- External links
Modern portfolio theory (MPT), or mean-variance analysis, is a mathematical framework for assembling a portfolio of assets such that the expected return is maximized for a given level of risk. It is a formalization and extension of diversification in investing, the idea that owning different kinds of financial assets is less risky than owning only one type. Its key insight is that an asset's risk and return should not be assessed by itself, but by how it contributes to a portfolio's overall risk and return. It uses the variance of asset prices as a proxy for risk [1].
Economist Harry Markowitz introduced MPT in a 1952 essay,[2] for which he was later awarded a Nobel Prize in Economics.
Mathematical model
Risk and expected return
MPT assumes that investors are risk averse, meaning that given two portfolios that offer the same expected return, investors will prefer the less risky one. Thus, an investor will take on increased risk only if compensated by higher expected returns. Conversely, an investor who wants higher expected returns must accept more risk. The exact trade-off will be the same for all investors, but different investors will evaluate the trade-off differently based on individual risk aversion characteristics. The implication is that a rational investor will not invest in a portfolio if a second portfolio exists with a more favorable risk-expected return profile – i.e., if for that level of risk an alternative portfolio exists that has better expected returns.
Under the model:
- Portfolio return is the proportion-weighted combination of the constituent assets' returns.
- Portfolio volatility is a function of the correlations ρij of the component assets, for all asset pairs (i, j).
In general:
- Expected return:
whereis the return on the portfolio,
is the return on asset i and
is the weighting of component asset
(that is, the proportion of asset "i" in the portfolio).
- Portfolio return variance:
,
whereis the (sample) standard deviation of the periodic returns on an asset, and
is the correlation coefficient between the returns on assets i and j. Alternatively the expression can be written as:
,
wherefor
, or
,
whereis the (sample) covariance of the periodic returns on the two assets, or alternatively denoted as
,
or
.
- Portfolio return volatility (standard deviation):
For a two asset portfolio:
- Portfolio return:
- Portfolio variance:
For a three asset portfolio:
- Portfolio return:
- Portfolio variance:
Diversification
An investor can reduce portfolio risk simply by holding combinations of instruments that are not perfectly positively correlated (correlation coefficient 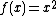 ). In other words, investors can reduce their exposure to individual asset risk by holding a diversified portfolio of assets. Diversification may allow for the same portfolio expected return with reduced risk. The mean-variance framework for constructing optimal investment portfolios was first posited by Markowitz and but has since been reinforced and improved by other economists and mathematicians who went on to account for the limitations of the framework.
). In other words, investors can reduce their exposure to individual asset risk by holding a diversified portfolio of assets. Diversification may allow for the same portfolio expected return with reduced risk. The mean-variance framework for constructing optimal investment portfolios was first posited by Markowitz and but has since been reinforced and improved by other economists and mathematicians who went on to account for the limitations of the framework.
If all the asset pairs have correlations of 0—they are perfectly uncorrelated—the portfolio's return variance is the sum over all assets of the square of the fraction held in the asset times the asset's return variance (and the portfolio standard deviation is the square root of this sum).
If all the asset pairs have correlations of 1—they are perfectly positively correlated—then the portfolio return’s standard deviation is the sum of the asset returns’ standard deviations weighted by the fractions held in the portfolio. For given portfolio weights and given standard deviations of asset returns, the case of all correlations being 1 gives the highest possible standard deviation of portfolio return.
Efficient frontier with no risk-free asset
{{main article|Efficient frontier}}{{see also|Portfolio optimization}}This graph shows expected return (vertical) versus standard deviation. This is called the 'risk-expected return space.' Every possible combination of risky assets, can be plotted in this risk-expected return space, and the collection of all such possible portfolios defines a region in this space. The left boundary of this region is a hyperbola,[3] and the upper edge of this region is the efficient frontier in the absence of a risk-free asset (sometimes called "the Markowitz bullet"). Combinations along this upper edge represent portfolios (including no holdings of the risk-free asset) for which there is lowest risk for a given level of expected return. Equivalently, a portfolio lying on the efficient frontier represents the combination offering the best possible expected return for given risk level. The tangent to the hyperbola at the tangency point indicates the best possible capital allocation line (CAL).
Matrices are preferred for calculations of the efficient frontier.
In matrix form, for a given "risk tolerance"
, the efficient frontier is found by minimizing the following expression:
where
is a vector of portfolio weights and
(The weights can be negative, which means investors can short a security.);
is the covariance matrix for the returns on the assets in the portfolio;
is a "risk tolerance" factor, where 0 results in the portfolio with minimal risk and
results in the portfolio infinitely far out on the frontier with both expected return and risk unbounded; and
is a vector of expected returns.
is the variance of portfolio return.
is the expected return on the portfolio.
The above optimization finds the point on the frontier at which the inverse of the slope of the frontier would be q if portfolio return variance instead of standard deviation were plotted horizontally. The frontier in its entirety is parametric on q.
Harry Markowitz developed a specific procedure for solving the above problem, called the critical line algorithm[3], that can handle additional linear constraints, upper and lower bounds on assets, and which is proved to work with a semi-positive definite covariance matrix. Examples of implementation of the critical line algorithm exist in Visual Basic for Applications[4], in JavaScript[5] and in a few other languages.
Also, many software packages, including MATLAB, Microsoft Excel, Mathematica and R, provide generic optimization routines so that using these for solving the above problem is possible, with potential caveats (poor numerical accuracy, requirement of positive definiteness of the covariance matrix...).
An alternative approach to specifying the efficient frontier is to do so parametrically on the expected portfolio return
This version of the problem requires that we minimize
subject to
for parameter
. This problem is easily solved using a Lagrange multiplier.
Two mutual fund theorem
One key result of the above analysis is the two mutual fund theorem.[6] This theorem states that any portfolio on the efficient frontier can be generated by holding a combination of any two given portfolios on the frontier; the latter two given portfolios are the "mutual funds" in the theorem's name. So in the absence of a risk-free asset, an investor can achieve any desired efficient portfolio even if all that is accessible is a pair of efficient mutual funds. If the location of the desired portfolio on the frontier is between the locations of the two mutual funds, both mutual funds will be held in positive quantities. If the desired portfolio is outside the range spanned by the two mutual funds, then one of the mutual funds must be sold short (held in negative quantity) while the size of the investment in the other mutual fund must be greater than the amount available for investment (the excess being funded by the borrowing from the other fund).
Risk-free asset and the capital allocation line
{{main article|Capital allocation line}}The risk-free asset is the (hypothetical) asset that pays a risk-free rate. In practice, short-term government securities (such as US treasury bills) are used as a risk-free asset, because they pay a fixed rate of interest and have exceptionally low default risk. The risk-free asset has zero variance in returns (hence is risk-free); it is also uncorrelated with any other asset (by definition, since its variance is zero). As a result, when it is combined with any other asset or portfolio of assets, the change in return is linearly related to the change in risk as the proportions in the combination vary.
When a risk-free asset is introduced, the half-line shown in the figure is the new efficient frontier. It is tangent to the hyperbola at the pure risky portfolio with the highest Sharpe ratio. Its vertical intercept represents a portfolio with 100% of holdings in the risk-free asset; the tangency with the hyperbola represents a portfolio with no risk-free holdings and 100% of assets held in the portfolio occurring at the tangency point; points between those points are portfolios containing positive amounts of both the risky tangency portfolio and the risk-free asset; and points on the half-line beyond the tangency point are leveraged portfolios involving negative holdings of the risk-free asset (the latter has been sold short—in other words, the investor has borrowed at the risk-free rate) and an amount invested in the tangency portfolio equal to more than 100% of the investor's initial capital. This efficient half-line is called the capital allocation line (CAL), and its formula can be shown to be
In this formula P is the sub-portfolio of risky assets at the tangency with the Markowitz bullet, F is the risk-free asset, and C is a combination of portfolios P and F.
By the diagram, the introduction of the risk-free asset as a possible component of the portfolio has improved the range of risk-expected return combinations available, because everywhere except at the tangency portfolio the half-line gives a higher expected return than the hyperbola does at every possible risk level. The fact that all points on the linear efficient locus can be achieved by a combination of holdings of the risk-free asset and the tangency portfolio is known as the one mutual fund theorem,[6] where the mutual fund referred to is the tangency portfolio.
Asset pricing
The above analysis describes optimal behavior of an individual investor. Asset pricing theory builds on this analysis in the following way. Since everyone holds the risky assets in identical proportions to each other—namely in the proportions given by the tangency portfolio—in market equilibrium the risky assets' prices, and therefore their expected returns, will adjust so that the ratios in the tangency portfolio are the same as the ratios in which the risky assets are supplied to the market. Thus relative supplies will equal relative demands. MPT derives the required expected return for a correctly priced asset in this context.
Systematic risk and specific risk
Specific risk is the risk associated with individual assets - within a portfolio these risks can be reduced through diversification (specific risks "cancel out"). Specific risk is also called diversifiable, unique, unsystematic, or idiosyncratic risk. Systematic risk (a.k.a. portfolio risk or market risk) refers to the risk common to all securities—except for selling short as noted below, systematic risk cannot be diversified away (within one market). Within the market portfolio, asset specific risk will be diversified away to the extent possible. Systematic risk is therefore equated with the risk (standard deviation) of the market portfolio.
Since a security will be purchased only if it improves the risk-expected return characteristics of the market portfolio, the relevant measure of the risk of a security is the risk it adds to the market portfolio, and not its risk in isolation.
In this context, the volatility of the asset, and its correlation with the market portfolio, are historically observed and are therefore given. (There are several approaches to asset pricing that attempt to price assets by modelling the stochastic properties of the moments of assets' returns - these are broadly referred to as conditional asset pricing models.)
Systematic risks within one market can be managed through a strategy of using both long and short positions within one portfolio, creating a "market neutral" portfolio. Market neutral portfolios, therefore, will be uncorrelated with broader market indices.
Capital asset pricing model
{{main article|Capital asset pricing model}}The asset return depends on the amount paid for the asset today. The price paid must ensure that the market portfolio's risk / return characteristics improve when the asset is added to it. The CAPM is a model that derives the theoretical required expected return (i.e., discount rate) for an asset in a market, given the risk-free rate available to investors and the risk of the market as a whole. The CAPM is usually expressed:
- β, Beta, is the measure of asset sensitivity to a movement in the overall market; Beta is usually found via regression on historical data. Betas exceeding one signify more than average "riskiness" in the sense of the asset's contribution to overall portfolio risk; betas below one indicate a lower than average risk contribution.
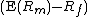 is the market premium, the expected excess return of the market portfolio's expected return over the risk-free rate.
is the market premium, the expected excess return of the market portfolio's expected return over the risk-free rate.
The derivation is as follows:
(1) The incremental impact on risk and expected return when an additional risky asset, a, is added to the market portfolio, m, follows from the formulae for a two-asset portfolio. These results are used to derive the asset-appropriate discount rate.
- Updated market portfolio's risk =
Hence, risk added to portfolio =but since the weight of the asset will be relatively low,i.e. additional risk =
- Market portfolio's expected return =
Hence additional expected return =(2) If an asset, a, is correctly priced, the improvement in its risk-to-expected return ratio achieved by adding it to the market portfolio, m, will at least match the gains of spending that money on an increased stake in the market portfolio. The assumption is that the investor will purchase the asset with funds borrowed at the risk-free rate,
; this is rational if
.
Thus:i.e. :i.e. :is the "beta",
return— the covariance between the asset's return and the market's return divided by the variance of the market return— i.e. the sensitivity of the asset price to movement in the market portfolio's value.
This equation can be estimated statistically using the following regression equation:
where αi is called the asset's alpha, βi is the asset's beta coefficient and SCL is the security characteristic line.
Once an asset's expected return,  , is calculated using CAPM, the future cash flows of the asset can be discounted to their present value using this rate to establish the correct price for the asset. A riskier stock will have a higher beta and will be discounted at a higher rate; less sensitive stocks will have lower betas and be discounted at a lower rate. In theory, an asset is correctly priced when its observed price is the same as its value calculated using the CAPM derived discount rate. If the observed price is higher than the valuation, then the asset is overvalued; it is undervalued for a too low price.
, is calculated using CAPM, the future cash flows of the asset can be discounted to their present value using this rate to establish the correct price for the asset. A riskier stock will have a higher beta and will be discounted at a higher rate; less sensitive stocks will have lower betas and be discounted at a lower rate. In theory, an asset is correctly priced when its observed price is the same as its value calculated using the CAPM derived discount rate. If the observed price is higher than the valuation, then the asset is overvalued; it is undervalued for a too low price.
Criticisms
Despite its theoretical importance, critics of MPT question whether it is an ideal investment tool, because its model of financial markets does not match the real world in many ways.[7][1]
The risk, return, and correlation measures used by MPT are based on expected values, which means that they are mathematical statements about the future (the expected value of returns is explicit in the above equations, and implicit in the definitions of variance and covariance). In practice, investors must substitute predictions based on historical measurements of asset return and volatility for these values in the equations. Very often such expected values fail to take account of new circumstances that did not exist when the historical data were generated.[8]
More fundamentally, investors are stuck with estimating key parameters from past market data because MPT attempts to model risk in terms of the likelihood of losses, but says nothing about why those losses might occur. The risk measurements used are probabilistic in nature, not structural. This is a major difference as compared to many engineering approaches to risk management.
{{quote|Options theory and MPT have at least one important conceptual difference from the probabilistic risk assessment done by nuclear power [plants]. A PRA is what economists would call a structural model. The components of a system and their relationships are modeled in Monte Carlo simulations. If valve X fails, it causes a loss of back pressure on pump Y, causing a drop in flow to vessel Z, and so on.
But in the Black–Scholes equation and MPT, there is no attempt to explain an underlying structure to price changes. Various outcomes are simply given probabilities. And, unlike the PRA, if there is no history of a particular system-level event like a liquidity crisis, there is no way to compute the odds of it. If nuclear engineers ran risk management this way, they would never be able to compute the odds of a meltdown at a particular plant until several similar events occurred in the same reactor design.
|Douglas W. Hubbard, 'The Failure of Risk Management', p. 67, John Wiley & Sons, 2009. {{ISBN|978-0-470-38795-5}}|source=}}
Mathematical risk measurements are also useful only to the degree that they reflect investors' true concerns—there is no point minimizing a variable that nobody cares about in practice. In particular, variance is a symmetric measure that counts abnormally high returns as just as risky as abnormally low returns. The psychological phenomenon of loss aversion is the idea that investors are more concerned about losses than gains, meaning that our intuitive concept of risk is fundamentally asymmetric in nature. There many other risk measures (like coherent risk measures) might better reflect investors' true preferences.
Modern portfolio theory has also been criticized because it assumes that returns follow a Gaussian distribution. Already in the 1960s, Benoit Mandelbrot and Eugene Fama showed the inadequacy of this assumption and proposed the use of stable distributions instead. Stefan Mittnik and Svetlozar Rachev presented strategies for deriving optimal portfolios in such settings.[9][10][11] More recently, financial economist Nassim Nicholas Taleb has also criticized modern portfolio theory on this ground, writing:{{quote|After the stock market crash (in 1987), they rewarded two theoreticians, Harry Markowitz and William Sharpe, who built beautifully Platonic models on a Gaussian base, contributing to what is called Modern Portfolio Theory. Simply, if you remove their Gaussian assumptions and treat prices as scalable, you are left with hot air. The Nobel Committee could have tested the Sharpe and Markowitz models—they work like quack remedies sold on the Internet—but nobody in Stockholm seems to have thought about it.|[12]{{rp|p.277}}}}
Contrarian/Value investors don't buy into Modern Portfolio Theory as it depends on the Efficient Market Hypothesis and conflates fluctuations in shareprice with "risk". This risk is only an opportunity to buy or sell assets at attractive prices inasmuch as it suits one's book.[13]
Extensions
Since MPT's introduction in 1952, many attempts have been made to improve the model, especially by using more realistic assumptions.
Post-modern portfolio theory extends MPT by adopting non-normally distributed, asymmetric, and fat-tailed measures of risk[14]. This helps with some of these problems, but not others.
Black-Litterman model optimization is an extension of unconstrained Markowitz optimization that incorporates relative and absolute 'views' on inputs of risk and returns from financial experts. With the advances in Artificial Intelligence, other information such as market sentiment and financial knowledge can be incorporated automatically to the 'views'[15].
Connection with rational choice theory
Modern portfolio theory is inconsistent with main axioms of rational choice theory, most notably with monotonicity axiom, stating that, if investing into portfolio X will, with probability one, return more money than investing into portfolio Y, then a rational investor should prefer X to Y. In contrast, modern portfolio theory is based on a different axiom, called variance aversion,[16]
and may recommend to invest into Y on the basis that it has lower variance. Maccheroni et al.[17] described choice theory which is the closest possible to the modern portfolio theory, while satisfying monotonicity axiom. Alternatively, mean-deviation analysis[18]
is a rational choice theory resulting from replacing variance by an appropriate deviation risk measure.
Other applications
In the 1970s, concepts from MPT found their way into the field of regional science. In a series of seminal works, Michael Conroy {{Citation needed|date=February 2011}} modeled the labor force in the economy using portfolio-theoretic methods to examine growth and variability in the labor force. This was followed by a long literature on the relationship between economic growth and volatility.[19]
More recently, modern portfolio theory has been used to model the self-concept in social psychology. When the self attributes comprising the self-concept constitute a well-diversified portfolio, then psychological outcomes at the level of the individual such as mood and self-esteem should be more stable than when the self-concept is undiversified. This prediction has been confirmed in studies involving human subjects.[20]
Recently, modern portfolio theory has been applied to modelling the uncertainty and correlation between documents in information retrieval. Given a query, the aim is to maximize the overall relevance of a ranked list of documents and at the same time minimize the overall uncertainty of the ranked list.[21]
Project portfolios and other "non-financial" assets
Some experts apply MPT to portfolios of projects and other assets besides financial instruments.[22][23] When MPT is applied outside of traditional financial portfolios, some differences between the different types of portfolios must be considered.
- The assets in financial portfolios are, for practical purposes, continuously divisible while portfolios of projects are "lumpy". For example, while we can compute that the optimal portfolio position for 3 stocks is, say, 44%, 35%, 21%, the optimal position for a project portfolio may not allow us to simply change the amount spent on a project. Projects might be all or nothing or, at least, have logical units that cannot be separated. A portfolio optimization method would have to take the discrete nature of projects into account.
- The assets of financial portfolios are liquid; they can be assessed or re-assessed at any point in time. But opportunities for launching new projects may be limited and may occur in limited windows of time. Projects that have already been initiated cannot be abandoned without the loss of the sunk costs (i.e., there is little or no recovery/salvage value of a half-complete project).
Neither of these necessarily eliminate the possibility of using MPT and such portfolios. They simply indicate the need to run the optimization with an additional set of mathematically expressed constraints that would not normally apply to financial portfolios.
Furthermore, some of the simplest elements of Modern Portfolio Theory are applicable to virtually any kind of portfolio. The concept of capturing the risk tolerance of an investor by documenting how much risk is acceptable for a given return may be applied to a variety of decision analysis problems. MPT uses historical variance as a measure of risk, but portfolios of assets like major projects don't have a well-defined "historical variance". In this case, the MPT investment boundary can be expressed in more general terms like "chance of an ROI less than cost of capital" or "chance of losing more than half of the investment". When risk is put in terms of uncertainty about forecasts and possible losses then the concept is transferable to various types of investment.[22]
See also
- {{sectionlink|Outline of finance|Portfolio theory}}
- Bias ratio (finance)
- Black-Litterman model
- Intertemporal portfolio choice
- Investment theory
- Kelly criterion
- Marginal conditional stochastic dominance
- Mutual fund separation theorem
- Omega ratio
- Post-modern portfolio theory
- Sortino ratio
- Treynor ratio
- Two-moment decision models
References
2. ^{{cite journal |author=Markowitz, H.M. |title=Portfolio Selection |journal=The Journal of Finance |date=March 1952 |volume=7 |issue=1 |pages=77–91 |doi=10.2307/2975974 |jstor=2975974|url=http://onlinelibrary.wiley.com/doi/10.1111/j.1540-6261.1952.tb01525.x/full}}
3. ^{{cite journal |author=Markowitz, H.M. |title=The Optimization of a Quadratic Function Subject to Linear Constraints |journal=Naval Research Logistics Quarterly |volume=3 |issue=1–2 |date=March 1956 |pages=111–133 | doi=10.1002/nav.3800030110 }}
4. ^{{Cite book|title=Mean-Variance Analysis in Portfolio Choice and Capital Markets|last=Markowitz|first=Harry|publisher=Wiley|date=February 2000|isbn=978-1-883-24975-5|location=|pages=|quote=|via=}}
5. ^{{Cite web|url=https://github.com/lequant40/portfolio_allocation_js|title=PortfolioAllocation JavaScript library|website=github.com/lequant40|access-date=2018-06-13}}
6. ^1 2 Merton, Robert. "An analytic derivation of the efficient portfolio frontier," Journal of Financial and Quantitative Analysis 7, September 1972, 1851-1872.
7. ^{{cite journal|authors=Mahdavi Damghani B.|title=The Non-Misleading Value of Inferred Correlation: An Introduction to the Cointelation Model|journal=Wilmott Magazine|volume=2013|issue=67|pages=50–61|year=2013|doi=10.1002/wilm.10252}}
8. ^{{cite journal|last1=Low|first1=R.K.Y.|last2=Faff|first2=R.|last3=Aas|first3=K.|title=Enhancing mean–variance portfolio selection by modeling distributional asymmetries|journal=Journal of Economics and Business|volume=85|pages=49–72|date=2016|doi=10.1016/j.jeconbus.2016.01.003|url=http://espace.library.uq.edu.au/view/UQ:377912/UQ377912_OA.pdf}}
9. ^Rachev, Svetlozar T. and Stefan Mittnik (2000), Stable Paretian Models in Finance, Wiley, {{ISBN|978-0-471-95314-2}}.
10. ^Risk Manager Journal (2006), {{cite web |title=New Approaches for Portfolio Optimization: Parting with the Bell Curve — Interview with Prof. Svetlozar Rachev and Prof.Stefan Mittnik |url=https://statistik.econ.kit.edu/download/doc_secure1/RM-Interview-Rachev-Mittnik-EnglishTranslation.pdf}}
11. ^{{cite journal |last=Doganoglu |first=Toker |authorlink= |author2=Hartz, Christoph|author3=Mittnik, Stefan |year=2007 |title=Portfolio Optimization When Risk Factors Are Conditionally Varying and Heavy Tailed |journal=Computational Economics |volume=29 |issue= 3–4|pages=333–354 |doi=10.1007/s10614-006-9071-1 |url=http://publikationen.ub.uni-frankfurt.de/files/2101/06_24.pdf}}
12. ^Taleb, Nassim Nicholas (2007), The Black Swan: The Impact of the Highly Improbable, Random House, {{ISBN|978-1-4000-6351-2}}.
13. ^Benjamin Graham (1949) "The Intelligent Investor"
14. ^{{Cite journal|last=Stoyanov|first=Stoyan|last2=Rachev|first2=Svetlozar|last3=Racheva-Yotova |first3=Boryana|last4=Fabozzi|first4=Frank|date=2011|title=Fat-Tailed Models for Risk Estimation|url=https://www.econstor.eu/bitstream/10419/45631/1/659400324.pdf|journal=The Journal of Portfolio Management|issue=2|volume=37|pages=107–117|doi=10.3905/jpm.2011.37.2.107}}
15. ^{{Cite journal|last=Xing|first=Frank Z.|last2=Cambria|first2=Erik|last3=Welsch|first3=Roy E.|date=2018|title=Intelligent Asset Allocation via Market Sentiment Views|url=http://sentic.net/market-sentiment-views.pdf|journal=IEEE Computational Intelligence Magazine|volume=13|issue=4|pages=25–34|doi=10.1109/MCI.2018.2866727}}
16. ^Loffler, A. (1996). Variance Aversion Implies μ-σ2-Criterion. Journal of economic theory, 69(2), 532-539.
17. ^Maccheroni, F., Marinacci, M., Rustichini, A., Taboga, M. (2009).Portfolio selection with monotone mean-variance preferences. Mathematical Finance, 19(3), 487-521.
18. ^Grechuk, B., Molyboha, A., Zabarankin, M. (2012). [https://www.researchgate.net/publication/51036152_Mean-Deviation_Analysis_in_the_Theory_of_Choice Mean-deviation analysis in the theory of choice], Risk Analysis: An International Journal, 32(8), 1277-1292.
19. ^{{cite journal |last=Chandra |first=Siddharth |authorlink= |year=2003 |title=Regional Economy Size and the Growth-Instability Frontier: Evidence from Europe |journal=Journal of Regional Science |volume=43 |issue=1 |pages=95–122 |doi=10.1111/1467-9787.00291 |url=}}
20. ^{{cite journal |last=Chandra |first=Siddharth |authorlink= |author2=Shadel, William G. |year=2007 |title=Crossing disciplinary boundaries: Applying financial portfolio theory to model the organization of the self-concept |journal=Journal of Research in Personality |volume=41 |issue=2 |pages=346–373 |doi=10.1016/j.jrp.2006.04.007 |url=}}
21. ^{{cite web|author=Portfolio Theory of Information Retrieval July 11th, 2009 |url=http://web4.cs.ucl.ac.uk/staff/jun.wang/blog/2009/07/11/portfolio-theory/ |title=Portfolio Theory of Information Retrieval | Dr. Jun Wang's Home Page |publisher=Web4.cs.ucl.ac.uk |date=2009-07-11 |accessdate=2012-09-05}}
22. ^1 {{cite book |title=How to Measure Anything: Finding the Value of Intangibles in Business |last=Hubbard |first=Douglas |authorlink= |year=2007 |publisher=John Wiley & Sons |location=Hoboken, NJ |isbn=978-0-470-11012-6 |pages= |url= }}
23. ^{{cite web |title=Manufacturing Portfolio Theory | last=Sabbadini |first=Tony |year=2010 |publisher=International Institute for Advanced Studies in Systems Research and Cybernetics |url=http://www.sctracker.com/wp-content/uploads/Manufacturing-Portfolio-Theory-CFPv1.pdf}}
Further reading
- {{cite journal |last=Lintner |first=John |authorlink= |year=1965 |title=The Valuation of Risk Assets and the Selection of Risky Investments in Stock Portfolios and Capital Budgets |journal=The Review of Economics and Statistics |volume=47 |issue=1 |pages=13–39 |doi=10.2307/1924119|jstor=1924119 }}
- {{cite journal |last=Sharpe |first=William F. |authorlink= |year=1964 |title=Capital asset prices: A theory of market equilibrium under conditions of risk |journal=Journal of Finance |volume=19 |issue=3 |pages=425–442 |doi= 10.2307/2977928|jstor=2977928 }}
- {{cite journal |doi=10.2307/2296205 |last=Tobin |first=James |authorlink= |year=1958 |title=Liquidity preference as behavior towards risk |journal=The Review of Economic Studies |volume=25 |issue= 2|pages=65–86|jstor=2296205 }}
External links
{{Commons category|Portfolio theory}}- Macro-Investment Analysis, Prof. William F. Sharpe, Stanford University
- Portfolio Optimization, Prof. Stephen P. Boyd, Stanford University
- "New Approaches for Portfolio Optimization: Parting with the Bell Curve — Interview with Prof. Svetlozar Rachev and Prof.Stefan Mittnik" https://statistik.econ.kit.edu/download/doc_secure1/RM-Interview-Rachev-Mittnik-EnglishTranslation.pdf
- Amathus (Transjordan)
- Amathus, Transjordan
- Amathuxidia
- Amati Cars
- AMATI Double Bass
- Amatignak Island (Alaska)
- AMatikulu
- Amatikulu
- Amatil
- Amatillo
- Amati (ritual)
- Amati (Ritual)
- Amatitlania siquia
- Amatitlán Municipality
- Amati (typeface)
- Amati violin
- Amati violins
- 'Amat Kuhwely
- Amatl
- Amatlan Zapotec
- Amatlar
- Amatlán de los Reyes Municipality
- Amatlán Zapotec
- Amatlán Zapoteco language
- Amato (horse)