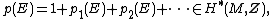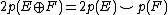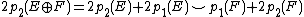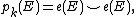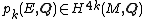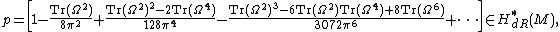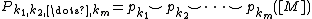1. Definition

2. Properties
     Pontryagin classes and curvature    Pontryagin classes of a manifold  

3. Pontryagin numbers
     Properties  

4. Generalizations

5. See also

6. References

7. External links

In mathematics, the Pontryagin classes, named for Lev Pontryagin, are certain characteristic classes. The Pontryagin class lies in cohomology groups with degree a multiple of four. It applies to real vector bundles.

Definition

Given a real vector bundle E over M, its k-th Pontryagin class p_k(E) is defined as

p_k(E) = p_k(E, Z) = (−1)^k c_2k(E ⊗ C) ∈ H^4k(M, Z),

where:

• c_2k(E ⊗ C) denotes the 2k-th Chern class of the complexification E ⊗ C = E ⊕ iE of E,
• H^4k(M, Z) is the 4k-cohomology group of M with integer coefficients.

The rational Pontryagin class p_k(E, Q) is defined to be the image of p_k(E) in H^4k(M, Q), the 4k-cohomology group of M with rational coefficients.

Properties

The total Pontryagin class

is (modulo 2-torsion) multiplicative with respect to

Whitney sum of vector bundles, i.e.,

for two vector bundles E and F over M. In terms of the individual Pontryagin classes p_k,

and so on.

The vanishing of the Pontryagin classes and Stiefel–Whitney classes of a vector bundle does not guarantee that the vector bundle is trivial. For example, up to vector bundle isomorphism, there is a unique nontrivial rank 10 vector bundle E₁₀ over the 9-sphere. (The clutching function for E₁₀ arises from the homotopy group π₈(O(10)) = Z/2Z.) The Pontryagin classes and Stiefel-Whitney classes all vanish: the Pontryagin classes don't exist in degree 9, and the Stiefel–Whitney class w₉ of E₁₀ vanishes by the Wu formula w₉ = w₁w₈ + Sq¹(w₈). Moreover, this vector bundle is stably nontrivial, i.e. the Whitney sum of E₁₀ with any trivial bundle remains nontrivial. {{Harv|Hatcher|2009|p=76}}

Given a 2k-dimensional vector bundle E we have

where e(E) denotes the Euler class of E, and denotes the cup product of cohomology classes.

Pontryagin classes and curvature

As was shown by Shiing-Shen Chern and André Weil around 1948, the rational Pontryagin classes

can be presented as differential forms which depend polynomially on the curvature form of a vector bundle. This Chern–Weil theory revealed a major connection between algebraic topology and global differential geometry.

For a vector bundle E over a n-dimensional differentiable manifold M equipped with a connection, the total Pontryagin class is expressed as

where Ω denotes the curvature form, and H*_dR(M) denotes the de Rham cohomology groups.{{Citation needed|date=July 2009}}

Pontryagin classes of a manifold

The Pontryagin classes of a smooth manifold are defined to be the Pontryagin classes of its tangent bundle.

Novikov proved in 1966 that if manifolds are homeomorphic then their rational Pontryagin classes p_k(M, Q) in H^4k(M, Q) are the same.

If the dimension is at least five, there are at most finitely many different smooth manifolds with given homotopy type and Pontryagin classes.

Pontryagin numbers

Pontryagin numbers are certain topological invariants of a smooth manifold. The Pontryagin number vanishes if the dimension of manifold is not divisible by 4. It is defined in terms of the Pontryagin classes of a manifold as follows:

Given a smooth 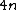-dimensional manifold M and a collection of natural numbers

such that ,

the Pontryagin number is defined by

where denotes the k-th Pontryagin class and [M] the fundamental class of M.

Properties

1. Pontryagin numbers are oriented cobordism invariant; and together with Stiefel-Whitney numbers they determine an oriented manifold's oriented cobordism class.
2. Pontryagin numbers of closed Riemannian manifolds (as well as Pontryagin classes) can be calculated as integrals of certain polynomials from the curvature tensor of a Riemannian manifold.
3. Invariants such as signature and 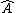-genus can be expressed through Pontryagin numbers. For the theorem describing the linear combination of Pontryagin numbers giving the signature see Hirzebruch signature theorem.

Generalizations

There is also a quaternionic Pontryagin class, for vector bundles with quaternion structure.

See also

• Chern–Simons form

References

• {{cite book

• {{Cite journal | last=Hatcher | first=Allen | author-link=Allen Hatcher | title=Vector Bundles & K-Theory | edition=2.1 | year=2009 | ref=harv | postscript= | url=http://www.math.cornell.edu/~hatcher/VBKT/VBpage.html}}

External links

• {{springer|title=Pontryagin class|id=p/p073750}}

• Trenkwalder Admira Amateure
• Tren Ligero
• Tren Loco
• Trenna
• Trenna Keating
• Trennert
• Trennert Type Foundry
• Trenoon
• Treno popolare
• Trenord
• Trenor William Park
• Treno Servizio Regionale
• Trenoweth
• Tren Patagonico
• Tren Patagónico
• Trentabank Reservoir Nature Reserve
• Trentadue
• Trent analogy
• Trent Ashby
• Trenta valley
• Trentavis Friday
• Trent Baker
• Trent Baretta
• Trent Barton
• Trent Bell