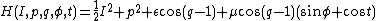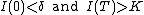- Background and statement
- See also
- References
In applied mathematics, Arnold diffusion is the phenomenon of instability of integrable Hamiltonian systems. The phenomenon is named after Vladimir Arnold who was the first to publish a result in the field in 1964.[1][2] More precisely, Arnold diffusion refers to results asserting the existence of solutions to nearly integrable Hamiltonian systems that exhibit a significant change in the action variables.
Background and statement
For integrable systems, one has the conservation of the action variables. According to the KAM theorem if we perturb an integrable system slightly, then many, though certainly not all, of the solutions of the perturbed system stay close, for all time, to the unperturbed system. In particular, since the action variables were originally conserved, the theorem tells us that there is only a small change in action for many solutions of the perturbed system.
However, as first noted in Arnold's paper,[1] there are nearly integrable systems for which there exist solutions that exhibit arbitrarily large growth in the action variables. More precisely, Arnold considered the example of nearly integrable Hamiltonian system with Hamiltonian
He showed that for this system, with any choice of 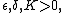 where
where  , there exists a
, there exists a 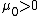 such that for all
such that for all 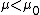 there is a solution to the system for which
there is a solution to the system for which
for some time 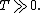
See also
- KAM theorem
- Nekhoroshev estimates
References
2. ^{{cite book|author1=Florin Diacu|author2=Philip Holmes|title=Celestial Encounters: The Origins of Chaos and Stability|url=https://books.google.com/books?id=26UtgDSw_MQC&pg=PA193|year=1996|publisher=Princeton University Press|isbn=0-691-00545-1|page=193}}
- Derek Wood
- Derek Wyatt
- Derelict
- Dereliction of duty
- Derelicts of Dialect
- Derelict soil
- De re militari
- Derendingen
- Derendingen, Switzerland
- De Republica
- De Re Publica
- De republica
- De re publica
- Derer et yndigt land
- Der er et yndigt Land
- Der er et yndigt land
- Der erlkonig
- De Rerum Natura
- De rerum natura
- Dere Street
- Derevlian
- De revolutionibus (Copernicus)
- De revolutionibus orbium celestium
- De revolutionibus orbium coelestium
- Der Ewige Jude