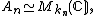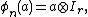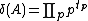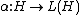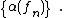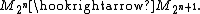- Definition
- Classification
- CAR algebra
- References
In mathematics, particularly in the theory of C*-algebras, a uniformly hyperfinite, or UHF, algebra is a C*-algebra that can be written as the closure, in the norm topology, of an increasing union of finite-dimensional full matrix algebras.
Definition
A UHF C*-algebra is the direct limit of an inductive system {An, φn} where each An is a finite-dimensional full matrix algebra and each φn : An → An+1 is a unital embedding. Suppressing the connecting maps, one can write
Classification
If
then rkn = kn + 1 for some integer r and
where Ir is the identity in the r × r matrices. The sequence ...kn|kn + 1|kn + 2... determines a formal product
where each p is prime and tp = sup {m | pm divides kn for some n}, possibly zero or infinite. The formal product δ(A) is said to be the supernatural number corresponding to A.[1] Glimm showed that the supernatural number is a complete invariant of UHF C*-algebras.[2] In particular, there are uncountably many isomorphism classes of UHF C*-algebras.
If δ(A) is finite, then A is the full matrix algebra Mδ(A). A UHF algebra is said to be of infinite type if each tp in δ(A) is 0 or ∞.
In the language of K-theory, each supernatural number
specifies an additive subgroup of Q that is the rational numbers of the type n/m where m formally divides δ(A). This group is the K0 group of A. [1]
CAR algebra
One example of a UHF C*-algebra is the CAR algebra. It is defined as follows: let H be a separable complex Hilbert space H with orthonormal basis fn and L(H) the bounded operators on H, consider a linear map
with the property that
The CAR algebra is the C*-algebra generated by
The embedding
can be identified with the multiplicity 2 embedding
Therefore, the CAR algebra has supernatural number 2∞.[3] This identification also yields that its K0 group is the dyadic rationals.
References
2. ^{{cite journal|last=Glimm|first=James G.|title=On a certain class of operator algebras|journal=Transactions of the American Mathematical Society|date=1 February 1960|volume=95|issue=2|pages=318–340|doi=10.1090/S0002-9947-1960-0112057-5|url=http://www.ams.org/journals/tran/1960-095-02/S0002-9947-1960-0112057-5/S0002-9947-1960-0112057-5.pdf|accessdate=2 March 2013}}
3. ^{{cite book|last=Davidson|first=Kenneth|authorlink=Kenneth Davidson (mathematician)|title=C*-Algebras by Example|year=1997|publisher=Fields Institute|isbn=0-8218-0599-1|pages=166, 218–219, 234}}
- List of Sites of Special Scientific Interest in Denbighshire
- List of Sites of Special Scientific Interest in Flintshire
- List of Sites of Special Scientific Interest in Gwynedd
- List of Sites of Special Scientific Interest in Isle of Anglesey
- List of Sites of Special Scientific Interest in Merthyr Tydfil
- List of Sites of Special Scientific Interest in Monmouthshire
- List of Sites of Special Scientific Interest in Neath Port Talbot
- List of Sites of Special Scientific Interest in Newport
- List of Sites of Special Scientific Interest in Pembrokeshire
- List of Sites of Special Scientific Interest in Powys
- List of Sites of Special Scientific Interest in Rhondda Cynon Taf
- List of Sites of Special Scientific Interest in Swansea
- List of Sites of Special Scientific Interest in Torfaen
- List of Sites of Special Scientific Interest in Vale of Glamorgan
- List of Sites of Special Scientific Interest in Wrexham
- List of sites on the Queensland Heritage Register in Toowoomba
- List of sites on the Queensland Heritage Register in Warwick
- List of sitting judges of the Supreme Court of India
- List of SIU Edwardsville Cougars men's basketball head coaches
- List of six-eyed spiders
- List of six eyed spiders
- List of Six Flags Fiesta Texas attractions
- List of six-man football fields in Texas
- List of six-man football stadiums in Texas
- List of Six Nations Championship hat-tricks