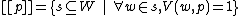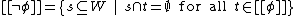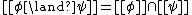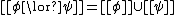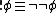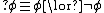1. Basic notions

2. References

3. Further reading

Basic notions

The essential notion in inquisitive semantics is that of an inquisitive proposition.

• An information state (alternately a classical proposition) is a set of possible worlds.
• An inquisitive proposition is a nonempty downward closed set of information states.

Inquisitive propositions encode informational content via the region of logical space which their information states cover. For instance, consider a simple inquisitive proposition which contains only a singleton information state {{math|{w} }} and the empty set . This inquisitive proposition conveys the information that the actual world must be {{math|{w}}}. In this respect, inquisitive propositions aren't very different from classical propositions, which also convey information by carving out a region of logical space. However, inquisitive propositions differ from classical ones in that they also convey inquisitive content by offering different avenues which one can take in refining their information. These avenues are provided by the maximal information states of an inquisitive proposition, which we call its alternatives. An inquisitive proposition can be thought of as raising the issue of which of its alternatives contains the actual world.

• Let P be an inquisitive proposition. Then s is an alternative of P iff s is a maximal element of P.

To see how inquisitive propositions work, let's look at two brief examples. Consider the inquisitive proposition P which contains two singleton information states {{math|{w₁} }} and {{math|{w₂} }}, as well as the empty set . P conveys the information that the actual world must either be w₁ or w₂, but it also raises the issue of which of those two ways the world actually is. Contrast this with the inquisitive proposition Q which consists of the information state {{math|{w₁, w₂} }} and all of its subsets. This inquisitive proposition conveys the same informational content as P, but it differs in its inquisitive content. Since Q contains only a single maximal information state, it offers only a single avenue for refining its information, and therefore doesn't raise any non-trivial issues.

We can isolate the informational content of an inquisitive proposition by pooling its constituent information states as shown below.

• The informational content of an inquisitive proposition P is .

We will make use of inquisitive propositions in order to provide an alternative interpretation of the language of propositional logic. Since the set of inquisitive propositions ordered by the subset relation forms a Heyting algebra, we can use the inventory of basic algebraic operations as the basis of our semantics. For instance, for every proposition P we have a relative pseudocomplement which amounts to . Similarly, for any propositions P and Q we have a meet and a join which amount to and 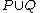respectively. Thus we can assign inquisitive propositions to formulas of as shown below.

Given a model 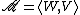where W is a set of possible worlds and V is a valuation function:

We will also use the operators ! and ? as abbreviations in the manner shown below.

Conceptually, the !-operator can be thought of as cancelling the issues raised by whatever it applies to while leaving its informational content untouched. For any formula , the inquisitive proposition expresses the same information as 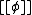, but it may differ in that it raises no nontrivial issues. For example, if 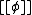is the inquisitive proposition P from a few paragraphs ago, then is the inquisitive proposition Q.

The ?-operator trivializes the information expressed by whatever it applies to, while converting information states which would establish that its issues are unresolvable into states which resolve it. This is very abstract, so let's consider another example. Imagine that logical space consists of four possible worlds, w₁, w₂, w₃, and w₄, and consider a formula such that 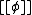contains {{math|{w₁} }}, {{math|{w₂} }}, and of course . This proposition conveys that the actual world is either w₁ or w₂ and raises the issue of which of those worlds it actually is. Therefore, the issue it raises would not be resolved if we learned that the actual world is in the information state {{math|{w₃, w₄} }}. Rather, learning this would show that the issue raised by our toy proposition is unresolvable. As a result, the proposition contains all the states of 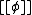, along with {{math|{w₃, w₄} }} and all of its subsets.

References

Further reading

• Ciardelli, Ivano; Groenendijk, Jeroen; and Roelofsen, Floris (2019) ‘’Inquisitive Semantics’’. Oxford University Press. {{ISBN|9780198814788}}
• https://projects.illc.uva.nl/inquisitivesemantics/

• Economy of abundance
• Economy of Afghanistan
• Economy of africa
• Economy of Africa
• Economy of Albania
• Economy of Alberta
• Economy of American Samoa
• Economy of Andorra
• Economy of Angola
• Economy of Anguilla
• Economy of Antarctica
• Economy of Armenia
• Economy of Aruba
• Economy of Asia
• Economy of Australia
• Economy of Austria
• Economy of Azerbaijan
• Economy of Bahrain
• Economy of Bangladesh
• Economy of Barbados
• Economy of Belgium
• Economy of Benin
• Economy of Bermuda
• Economy of Bhutan
• Economy of Birmingham